My Other Math Sites
Lessons From the Classroom
The Right Question Institute
From www.aish.com:
Isidore Rabi, winner of a Nobel Prize for physics, was once asked why he became a scientist. He replied: “My mother made me a scientist without ever knowing it. Every other child would come back from school and be asked, ‘What did you learn today?’ But my mother used to say, ‘Izzy, did you ask a good question today?’ That made the difference. Asking good questions made me into a scientist.”
A week ago my superintendent, principal, and 7 of us teachers attended a full day workshop The Right Question Institute in LA. Luz Santana and Dan Rothstein, authors of Make Just One Change, facilitated a worthwhile and engaging session, so I just want to share some highlights and my takeaways from it.
Highlights
(Some of these might be direct quotes. I’m just writing from my notes.)
Not knowing what to ask is the fundamental obstacle to participating and therefore to learning.
The skill of question formulation is the single most powerful renewable source of intellectual energy.
How can we easily develop students’ question formulation skills? It’s simple. But simple does not mean simplistic — it means doing it so everyone can access it.
Six components of the Question Formulation Technique (QFT):
A question focus — the teacher gives a prompt related to topic currently being covered in class, prompt can be visual. It should be a statement or phrase and not as a question. The simpler, the better.
Producing questions — in small groups, students share questions that they have related to the question focus (prompt), one person records on large poster paper or whiteboard. But before starting, everyone is reminded of these rules:
Ask as many questions as you can.
Do not stop to discuss, judge, or answer any question.
Write down every question exactly as it is stated.
Change any statements into questions.
Categorizing questions as “open” or “closed.”
Prioritizing questions — choose 3 most important questions from list, pay attention to the question focus.
Next steps — what’s one way you could use your priority questions?
Reflecting — what did you learn and how did you learn it?
Takeaways
Luz and Dan are really lovely people. Warm, hard-working, fun. Their book — and this Institute — mark the arrival of a twenty-year journey for them! (Arrived, yes. Settled, no.)
This task of having kids ask their own questions is not unlike Act 1 of a 3-Act math task that many of us are familiar with.
But the QFT process can be used — and is used — in a variety of academic disciplines and in communities outside of school. (Their journey actually began when they worked on a dropout prevention project and heard from the parents who were not coming to the school meetings because they “don’t even know what to ask.”)
This is another powerful structure that empowers students when asking questions becomes a natural tool for them. They think more critically because the QFT process helps them hone in on their questions. Dan and Luz categorize the learning of asking questions as going from divergent thinking to convergent thinking, then that last component of reflecting is metacognitive thinking.
Teachers and students will get better at implementing the QFT. It’s building classroom culture, so it takes time.
We’re doing this already with our students to some degree with varying expertise. Maybe we just need to be more intentional about it. Give it a name.
If not, perhaps Make Just One Change.
What Our Students Thought of Ability Grouping
A month ago I wrote about Ability Grouping and within that week asked my students for their thoughts, but I've been swamped with work to share any sooner. My teaching assignment was:
Geometry: 32 students. All were 8th graders, largest group we'd ever had, 45% more than the year before. A handful of them were not ready for the rigors of this high-school equivalent course; it was more due to scheduling that they ended up here. (We have about 200 kids in the junior high, 2 sections of each level, so it's not easy to be flexible with our schedule.)
Algebra 1: 38 students. Ten were 7th graders, the rest were 8th graders, and 3 of the 8th graders worked independently out of the Algebra Readiness textbook at midyear as they struggled to continue on, but they joined the rest of the class for all problem-solving group tasks which happened about twice a week.
Math 6: 69 students in two classes, but 2 of them were in RSP for math, so I only saw them on Fridays.
My colleague Erin helped me give her students the same survey questions. I don't know her exact roster counts, but she taught two classes of Pre-Algebra (all were 7th graders) and one class of Algebra Readiness to 8th graders. I began by telling the kids a little bit about ability grouping at our school, that it existed in grade 7 and grade 8, that we tried to place them based on several measures (grades, work habits, CST scores, benchmark tests). I did not tell them how I felt previously or presently about ability grouping, but I wanted to learn what they'd thought. I gave each student this strip of paper and asked them to check one box.I then added these three questions: I'm going to summarize what boxes the kids checked for both parts above like this:
From the Geometry kids (there was some city-level academic competition going on that day, so more absences than usual):
From the Algebra 1 kids:
From the Algebra Readiness kids:
From the Pre-Algebra kids:
From the 6th Graders:
So, about 83% (151/183) of the kids said YES to ability grouping. Sure, there were a few kids who seemed to have conflicting responses by checking NO to the first question but had more YES responses with the 3 questions that followed, and vice versa. I don't know. I do know this: Erin and I really love our students and love what we do. She has a math degree, I do not, but we both love problem solving and are proud math enthusiasts. She let me talk her into going for a week-long training in Palo Alto to jump start — actually it was more of a revival of — a Math Teachers' Circle in our area. I want to believe that Erin and I made a difference in how our students felt about their learning of mathematics. Doug left a comment on my Ability Grouping post. His last paragraph strikes the perfect note of what I want to say right now:
I wonder if the bigger problem is teaching students to not be so concerned with who is "ahead" of whom. Maybe the problem has less to do with what class you put the students in, and more to do with how you treat them once they get there. We need to foster the growth mindset. Maybe our fixed-ability mindset (ala Carol Dweck etc.) primes us to be unnaturally sensitive about placement. Most of us will live and die always knowing there is someone, lots of people, more competent/talented/accomplished than we are at everything we do. But we have to live for ourselves, and pursue what we care about, regardless. The bigger issue is making sure every classroom has a good teacher presenting quality material. Then it doesn't matter who is in what room with whom.
Thank you, Doug.
Playing With Math: Stories From Math Circles, Homeschoolers, and Passionate Teachers
Sue VanHattum's blog Math Mama Writes was one of the earliest math blogs I frequented. Sue and Shireen, Dan, Kate, and Sam were among the first people who showed me — through their passionate writing — that there was an online community where we may share the teaching and learning of mathematics in the classroom.
When I started this blog, Sue dropped in to leave a comment or two. Or five. We even talked on the phone, and she shared with me how her son got his name. I remember reading someone's post and wanting to leave a comment because I connected with the piece and its author in some small way, and inevitably I would find that Sue had already left a comment. This happened over and over again. I felt we were reading the same stuff; and the same stuff touched us in similar ways.
Then, within a year Sue asked if I would like one of my posts to appear in a book she was putting together.
So, it's with much love and honor that I get to help launch Sue's new book:
Playing With Math: Stories from Math Circles, Homeschoolers, and Passionate Teachers brings together the stories of over thirty authors who share their math enthusiasm with their communities, families, or students. After every chapter is a puzzle, game, or activity to get you and your kids playing with math too.
To know Sue is to know that she loves teaching and learning mathematics, and she loves writing, therefore this book — this work of consummate love - has to happen.
Playing With Math is really a collection of love stories because the authors, including yours truly, want to share something we're pretty crazy about. It's the stuff we do beyond the regular school day — we play with math after hours, at the dinner table, on a napkin at the coffee shop, with our own child or with a neighbor's child, at a family picnic, with our in-laws whom we don't even like.
So, today is the first day of our crowd-funding campaign to cover production costs. We're hoping to find support in our community of teachers and parents and math connoisseurs — a community of people whom I adore and respect. You can contribute anything from $1 to $1 billion. But for a contribution of $25, this wonderful book will be sent to you as soon as it's printed. Please see more details here.
Thank you so very much.
Mindset
I’m very grateful to be here at the 3-day MAP Conference in South San Francisco — finally got to meet Malcolm Swan whose lessons I’ve used with my students for many years. I was delighted to see this slide because we did this lesson and it was one of my favorites.
At the end of today's session, we were asked to share questions that we may have for tomorrow's panel of speakers. A teacher asked this question, and it stayed with me. He said something like this:
The students in the video [during Malcolm's talk] are awesome. But my students are not there. They are at zero. Not even zero, they are at negative something...
Negative?? Zero?? It made me sad to hear this. No matter how "low" his students are, no matter how out-of-control they appear, no matter how unmotivated they seem, they cannot be at level zero. That's impossible. We talk a lot about students' mindsets. I worry more about the teacher's mindset. Maybe this teacher didn't mean it the way I'd heard it; but I've heard teachers say directly to me, "My kids can't do that." How do we know that unless we give them a chance.
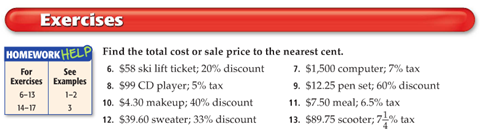
Discount and Sales Tax
Instead of doing the above exercises in our textbook, I had my 6th graders do this:
Shopping Contest at Target
Let’s pretend Target has a contest. The contest is for shoppers to find merchandise from their online store.
Contest rules
You must choose at least 10 different items.
The items must come from at least 5 different departments, such as footwear, kitchen, clothing, toys, etc.
You may buy more than 1 same item — you can buy 2 or more packs of athletic socks.
The winner
The winner is the shopper whose merchandise receipt totals exactly $500 or closest to it — without going over. They get to keep all the merchandise!
Your task
Phase 1: Go to Target’s website and find some items that you’ll want to get for yourself and your family. List the items and their original prices in the table provided separately.
Phase 2: The store manager (really, it’s your teacher) then announces the percent discounts for different categories of merchandise. Write these down below. Apply these discounts to your items and calculate the new sale prices.
Phase 3: The current tax rate for our city is 8%, so you must add this to your total. In this phase, you get to add or remove items on your shopping list to reach the target goal of $500 without going over.
I only did Phase 1 with the kids, my sub supposedly did Phases 2 and 3 with them. (I wanted to do the rest of the lesson with them when I return from NCTM — and after spring break — but they voted to continue the lesson with the sub as they were really into the task. I'll find out tomorrow when class resumes.)
Why this task
You can change everything about it. Shop somewhere else instead of Target.
Change the rules depending on time available and access to computers. Are there enough computers for each kid or do they need to work in groups of 2 or 3? My kids were in pairs. (We have enough laptops, but at least 30% of them have issues.)
I like the idea of not going over a certain amount — $500 in this case — instead of "whoever is closest to the target price" because I think it keeps the kids more reserved in their shopping spree. Students understand that if there were only 2 contestants, then the one with a final receipt total of $154 would win over the other with a $501 total.
Kids don't know what the exact discounts are until Phase 2, so this makes it a fun temporary secret. But they know to go over budget in Phase 1 because there will be discounts — not all departments have to have discounts either. Their totals in Phase 1 were in the $600 to $700 range because they also know that it'd be easier (faster) to remove items than add them later in Phase 3. They were also told that the discounts would be somewhat realistic, meaning Electronics will get a smaller percent discount, if any, than Clothing.
They'll become more aware of how much things cost — and how quickly they add up in the shopping cart.
Handout Discount and Sales Tax
Prices, Proportions, Percents
I was in Garden Grove with my son on Sunday, and he insisted that I try this smoothie place called Tastea. With Jamba Juice and Blenders and all the other juice bars around town, I was skeptical that this joint's concoctions would be anything different. He ordered a taro milk tea and I got a Thai tea, both with boba. Just one sip and I said to him, "Let's order another round! It's a long drive home!" Soooo delicious.
While there I saw a math lesson brewing, so I picked up their menu with prices. This is the lesson with my two classes of 6th graders.
Me: (I tell them about Tastea and how I wish it were closer.) Okay, let's start with something you might be more familiar with, Starbucks. I love that now I live within walking distance from one! Do you know the different sizes that they have there?
Class: (When I refer to "class," I don't mean the whole class, of course, but somebody in the class joins in on the conversation.) Tall, grande, venti.
M: Do you know exactly how much liquid each size holds? (They make various guesses. I bring out the 3 sizes that I got from Starbucks so they have a visual.) I normally order a tall mocha frappuccino, let's say the price is $3.50. Do you think the venti, which is twice the volume of the tall, would cost twice as much, or $7.00?
C: No.
M: Why not?
C: You normally get a better deal with a bigger size.
M: What do you mean by a "better deal"?
C: (All their answers show me that they understand the idea of more bang for your buck. Then finally someone says...) Lowest unit price!
M: Right! That's why so many families go to Costco. Buying in bulk normally saves us money because the item has the best unit price. Well, we're talking about Starbucks now, so buying more is a better deal, but drinking more is not so good for our body. Let's fill in this sheet. (I pass out this handout. And here’s the key.) How do we calculate unit price? What place value should we round it to? How do you write thirty-one-cents-per-ounce?
M: Tastea has three sizes: mini, gigantic, and even more. Their teas can also be purchased by the "partea jug," which holds a gallon. I've given you the prices of the 10-ounce minis for the three different types of drinks, your job is to figure out the prices of the other sizes. You'll work in small groups to figure out these out. So, do you think the gigantic will cost twice as much as the mini because it holds twice as much?
C: No. It'll cost less.
M: How much less? Well, that's your group's job to come up with the best estimate. We have Starbucks' prices for their three sizes, you could look at how they price their drinks. But here's the sweet deal for you. You and your group mates do the math that you need to, then write down your first estimation right here in this column. Bring your paper up to me (only the "captain's" paper), give me a few seconds to figure out the percentage that your estimation is off by, and I'll write it in this column and give you back your paper. What percent do you want to see, large or small? What if your estimation were the actual price — what percent would I write there?
I tell them that they could figure out the actual price of the drink if they knew how I calculated the percentage of error. So, work work work. Think think think. What makes sense? Oh, I remind them that the percentage does not indicate if their estimation is too high or too low. So, again, what makes sense?
We also note that prices generally end in a 0, 5, or 9. So, even if the calculation tells them the price should be $4.23, they might want to change that to $4.25 or $4.20.
When they bring up their paper again with the second estimation, all I do is write their estimation again in pen and circle it — this is so they can't change their answer and I know that I've seen it. I do NOT fill in the "Actual Price" column at this time because the groups are working at different rates, and in a crowded room, it's easy for kids to see each other's papers, even inadvertently, and the game of estimation is over if they saw the actual price beforehand.
They simply move on to the next size to make a first estimation again. We repeat the process.
When all groups are done with estimations for the first type of juice — smoothies — I tell them what the actual prices are.
Now, it's their turn to figure out the percentage of error. I give the groups about 10 minutes to do so without help from me. At the end of the 10 minutes, either there's at least one group that knows how to do so and can show it to the class, or no group knows how, then I'll walk them through the calculation by asking them questions to figure this out.
They continue in the same manner for the Slushy Freeze and Specialteas on page 2. This time hopefully they'll be able to work backward from the error percentage that I give them after their first estimation.
Reasons I'm proud of this lesson:
It's about proportions, but many priced items in real life are not directly proportional. The kids knew this coming in because they've been consumers.
We get to talk about business strategies that entice people to buy the larger sizes while still make a profit. (Starbucks calls it "tall" because it rhymes with "small," but clearly the word tall naturally elongates the imagination.)
Students get to make estimations throughout, but they know these aren't "wild-ass guesses." They start with the calculation of proportions and adjust the prices accordingly. They get to critique and argue with their group mates to come up with the best estimations.
I get kids to think about percentage in a context that they can wrap their heads around. And they want to know how because their second estimation could be dead on if they knew.
It's fun that the error percentage does not indicate if their estimation is too high or too low. A few groups do go farther in the wrong direction. Oh, well — good to learn that now.
It'd be fun for me to get Starbucks or Jamba Juice for the group with the lowest total in percentage errors.
Updated 04/05/14
I got some thoughtful reflections on this lesson, I'll just share two:
I learned how to work backwards with percentages and try to get the number spot on. I also learned how business would price things by dropping the price by the perfect amount. My number sense got a lot better from all the multiplying, dividing, and reasoning. It was very difficult, which I'm very happy about. The teamwork was probably the hardest part of the project. M and I are very competitive, and we got different answers a lot. I learned how to work together with others a lot better, and it doesn't move your team along to place blame and argue. I'm really grateful we did this project because it was very hard and worthwhile. It was a great use of three days!
I learned how to use different data to get answers. Also, we have to see a pattern. This Tastea assignment was really fun. I enjoyed it and look forward to another. Teamwork is really important even though people can't agree, you got to support it. If your group gets it wrong, but your answer was right, you can't blame someone or put them down because probably they will get some right for you. So always stay positive to your teammates and encourage them.
What the Kids Thought
Recently Dan Meyer asks Mathalicious which of these three questions is "real world"?
Karim Ani, founder of Mathalicious, and others have opined without consensus on this particular question and on the general notion of real-world vs. fake-world problems.
I wonder what my 8th grade geometry kids think of this question.
I give them Version A on a strip of paper and ask them to work on it alone for 5 minutes. I tell them that I'm interested in learning if they understand the question as is, therefore I'm not answering any clarifying questions about it. After the 5 minutes, I put them into random groups, and they work on the problem for another 10 minutes.
Then I show them Versions B and C and ask for their preference and reason for each version.
Version A: 18 likes, 14 dislikes
Highlighted reasons for LIKING:
It gives every detail you need to know. It tells you directly all of the information. It also seems easiest to solve.
It isn't as confusing as looking at fast-motion pictures of a circle and a square. Doing math is more exact than visual guessing. [Did he translate the animation to mean guessing?]
I am able to make my own diagram and I can try to solve an equation to find out the answer.
It is simple and not confusing. It allows me to think the way I want to and not be misled by a moving picture.
With the given information, you could construct the two shapes by working backwards.
There's enough given information to make the problem interesting and hard.
I like this one the most because you can actually read the problem and refer back to it.
I think it can be solved using an equation, and be solved more easily than B and C.
It's a more accurate way to find their areas and make them equal.
I like this version because I understand the problem.
Instead of a picture of it on paper, you have to visualize it in your head first.
You can get an exact answer. It is challenging.
Version B: 18 likes, 14 dislikes
Highlighted reasons for LIKING:
It would be cool to build the animation in GSP and solve it that way.
It's a lot more simple. It provides an image and idea of what it looks like.
I can visually see when they are equal. It will be easier to see when they are equal instead of having to do a load of math.
It is visual.
I feel I have a higher chance of answering the question with a right answer.
You can easily see when the shapes have the same area.
Version C: 15 likes, 17 dislikes
Highlighted reasons for LIKING:
It seems easier because you can just count the candies and see if they're equal.
Anyone can count how many candies there are, then subtract the extra space to get the correct area.
Some students like and dislike more than one version. My takeaway on their responses:
Version A
LIKES: (see above)
DISLIKES: Not understanding the question, or "I'm a visual learner, so I like Version B better."
Version B
LIKES: It's visual. It's easy.
DISLIKES: Too fast and hard to follow. One student, "The movement is distracting and confusing. I feel like it's too abrasive and violent. Math should be more elegant than this."
Version C
LIKES: You just count the number of candies. It's visual.
DISLIKES: Too fast to follow. It seems too easy. There's space between the candies. One student, "You can't get the exact answer... And the leftover space in one shape may be more than the leftover space in the other."
I collect all their papers before telling them which version I like. I like Version A for its simplicity. I'm curious if the stated question is enough information for them to understand. This student's reason nails it for me: "It allows me to think the way I want to and not be misled by a moving picture."
We all have students who struggle with word problems. I don't think this means we should give them fewer word problems. I think it means we should give them better word problems — ones that are written with just enough information and not embedded in contrived contexts that either confuse or insult the students. And for students who need help with the question, they get to hear an explanation from a classmate.
Version B is okay, but I don't want to start with it because I feel I'd be wasting a perfectly good question in Version A! I'd reach for a piece of string to explain this question, if needed. Version C gives me a headache.
At least one person in each group understands the question, and they do their best to make sense of it in just the short 10 minutes that we have. They're trying. And making mistakes.
Our whole-class discussion at this 8th grade level:
That "arbitrary" point P is pretty darn close to the middle of A and B. You can roughly tell from using a piece of string. Or you can tell from arriving at y2/(4pi) = x2/16 (where x is distance AP forming the square's perimeter, and y is distance PB forming the circle's circumference) — the denominators are almost the same.
Likewise, P cannot be at the center because pi doesn't reconcile nicely in the equation.
We can solve for x and y using some arbitrary distance AB, and we find y to be slightly shorter.
We can ask a related question: A circle's circumference and a square's perimeter are equal, what is the area enclosed by each? Kids can certainly think about optimization and do a little bit of calculation outside of a formal calculus class.
In addition to asking the students which versions they like, I also pose Dan's exact question to them: Which of these is a "real world" math problem? Or is none of them a real-world math problem?
Their answers vary as widely as those of math educators'. However, I find this correlation that doesn't surprise me: kids who like math more do not care if the problem is real-world or not.
This [Version C] is the most "real-world" solely because of the fact that it involves a material object which in this case is the candy. However, the thing you're solving for in this question is not very "real-world" at all. Personally, I don't care at all if a problem is "real-world" or not; I just like to solve problems.
If a problem didn't have to do with "real-world" I will still do it if I like it. It doesn't really matter.
I don't think any of these problems are "real world" math problems. I like how they make me think. But I don't think I need them in the "real world."
I wouldn't care if it is a real-world problem because I was there to learn. I think all versions can be a real-world problem because it can be needed in some situations.
I feel like all of these problems are real world... But honestly it doesn't matter at all to me. It doesn't matter if it's real world or not, it doesn't affect me wanting to solve the problem.
So, one from the kids.
Teaching Absolute Value
I know Common Core does not have absolute value in grade 8, but I'm teaching it anyway because we're still doing "algebra 1" this year. (A year ago Raymond Johnson looked into the inclusion of this topic in the different grade levels.) My 8th graders know that the absolute value of 5 is 5, and the absolute value of -5 is also 5. Some recall that it's the distance from 0 on the number line. We begin by solving a few of these: abs(x) = 4, abs(a) = 0, abs(w) = -3. A few trip up on the last one but recover quickly and move along.
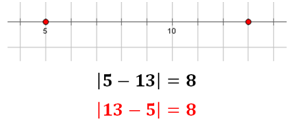
Me: What is the distance between these two points?
Class: Eight.
M: How did you get eight?
C: Subtract.
M: What about this one? The points are at -3 and 9.
C: The distance is twelve.
M: This one?
C: Thirty-two.
M: Good. Distance is always positive... How did you find the distance between the points again? What operation did you use?
C: Subtraction!
M: Then I'm going to add an equation below each number line showing subtraction. Is that okay?
M: So, the distance between 5 and 13 is 8. Then, what is the distance between 13 and 5?
C: Eight
M: Woah! It's the same? Meaning I can write the equation either way?
Kids agree that subtraction can be commutative when it's inside the absolute value bars because we’re just measuring distance. The distance from Johnny to Julie is the same distance as from Julie to Johnny. I'm not going to argue.
Me: Given two points, you can tell me the distance between them. So now I’m going to give you just one of the two points but tell you the distance between them, and you find the missing point x.
C: x is ten.
M: Yea, ten works. Let's try to read this open sentence. How would you say it?
C: x minus six... The absolute value of x minus six is four.
M: Hmmm. Oh, you say the words 'absolute value' because they're there. Let's try again without saying those words. Use the word 'distance' instead.
C: The distance of x minus six equals four.
M: Let me show you again the first one that I'd asked you. I remember just asking you, 'What is the distance between 5 and 13?' What did I not say even though it's there?
C: Minus.
M: Right. Let's not add stuff we don't need. You know naturally that finding distance implies subtraction. So, say the equation again.
C: The distance between x and six is four.
M: Or you could say...? Can we switch the points around?
C: The distance between six and some point x is four.
M: Alright. Is 10 the only answer for x? We are trying to find a point on the line that makes the equation true. So, let's use the number line to solve this. Because we know 6 is one of the points, let's locate it. We need to find the other point that would be a distance of 4 away from 6. So, it could be to the right of 6, or to the left of 6. Where does this put us at?
M: Oh, why isn't the point -6? I see a 'minus six' in the equation.
C: Remember, that minus is for subtracting. We need it there to find distance.
M: I remember. We need it.
We do a few more of these. Enough to bore us, need something new.
M: Let's try this.
C: No subtraction sign.
M: And you said we needed it. Then create it. Make it happen without changing the problem of course.
C: Change it to minus minus...
M: What does this problem say now?
C: The distance between x and negative eight equals five.
We do a few more of these. Enough to bore us, need something new again.
M: What about this?
M: Nothing terribly exciting. The other point(s) that we find is now worth 2x, so we just need to solve for x.
Then we do a batch of these:
Hey, what about these, where there's more stuff stuck around the absolute value quantity. Oh, we just need to first isolate the absolute value, then it's business as usual.
We spend the next whole class using Desmos to explore the shifts/changes to the parent absolute value function. Students need to write down their predictions first before graphing. One student was very excited when she got the V-shape to turn upside down.We discuss some real-life scenarios that may involve absolute values: margins of error, ranges of measurements: distances, scores, speed, temperatures, pH levels, elevations, etc.
Not proud to admit that I spent a lot of hours in college playing pool instead of studying, but never once did I associate the path of the ball as an absolute value function. Consider me odd if I always thought of angles instead.
Solving absolute value inequalities start similarly enough. Kids know from graphing inequalities that there's "shading" involved. They also know the difference between open and closed points. So I just have the kids use their thumb and forefinger to indicate the distance between the two points, then if the inequality says less than [or equal to], then it's natural to pinch their fingers closer together, indicating that the region inside the points need to be shaded.
The textbook will tell kids to set up the "two cases" to solve these inequalities (same thing with equations).
Then, kids are asked to graph the solution. But if kids learned to solve using the number line itself, then there would be nothing to memorize because they learned distance way back when they started learning to crawl. And since the graph shows the solution, then writing what that solution is is easy because it matches the graph. Like below, x lives on the green line between -8 and -2, being ≥ -8 and ≤ -2.
For greater than inequalities, the student would naturally spread his fingers apart to indicate shading outside of the points.
I don't know why there seems to be a lot of rules when learning to solve absolute value equations — which inequality sign for when it's and or when it's or.
Classroom Management
Give or take, scenes from my classroom last week:
A walks into class, talking at full volume until someone shushes her and points to the obvious math talk on the board.
B slouches in his desk, head barely above seat-back.
C decides to dump out contents of his binder to find the math paper from 24 hours ago.
D talks while I’m talking.
E and F are talking while someone else is sharing.
G and H are playing footsie; H is better at this.
Someone lets out a shockingly loud fart — we all look at row 7, seat 5 because the occupant is giggling and beaming proudly.
I continually scans the room like she’s seeing it for the first time.
J asks to use the restroom when there are fewer than ten minutes left of class.
K and L try to talk to each other half way across the room.
M makes squishy noises with his water bottle.
N taps his pencil.
O clicks his pen.
P and Q… well, they’re just minding themselves.
R blurts out, “I already got the answer!”
S needs to borrow a pencil from classmate for the 95th day of school.
T volunteers, “Yes, I’m very bright. I’m a genius. But I need help with section nine four.”
U returns my look with a look of what-Mrs.Win-?-I’m-doing-my-work-see-?-hehe-okay-I’ll-do-my-work-now.
V yells at person sitting in front of her, “Stop pushing your desk into me!!”
W walks across room to get a drink of water. Five sets of eyes follow W and then at me to see my reaction.
X asks out of the blue, “Have you eaten at that Korean place, Mrs. Win? It’s so good.”
Y sticks out his foot to trip Z as he walks by.
A through N immediately engage in lively conversations just as I say, “I need you to take out a piece of graph paper.” So, I have to say, “Guys! You don’t have to talk just to get out a piece of paper!”
O through Z immediately engage in lively conversations just as I say, “Make sure your name is on the paper.”
I may only talk about classroom management with your understanding that my own classroom is sometimes chaotic, sometimes louder than it should be, sometimes messy — but somewhere in this soup of chaos, noise, and mess, I have to believe that there is learning of mathematics. More so on some days.
I can’t help but draw parallels between teaching and parenting because both roles have defined me. Their enormous responsibilities have brought out the best and worst in me. It’s easy to love children. It’s much harder to discipline them. A wise colleague once reminded our staff that discipline is not a dirty word — to discipline means to teach. And I think teaching is the purest form of love because teaching is sharing.
Classroom management is used interchangeably as classroom discipline, and that’s okay. It’s all part of classroom teaching. I’ve been around long enough to see teachers leave the profession because they lacked “classroom control.” Nothing in teacher school adequately prepares you for this. No manual outlines what to do when a kid cusses you out. Step 1: remain calm. Step 2: breathe deeply and count to 10. Step 3: fuck this shit and find another job.
I bought this hardcover book long ago.
I’m sure it’s full of good intentions and sound advice. (Serendipitous that 20 years later the author Randall S. Sprick enters my life again when our school currently adopts his CHAMPS program.) But it’s really hard to see classroom management in action from reading a book or a blog post. It’s ideal to directly observe a teacher and her students, and not just for a day or two, but over a period of time. The classroom culture is undeniably real and one has to be in it to fully appreciate and honor this culture. I know there’s a thing called student teaching where one is immersed in a real classroom for a semester. But I swear to God, the kids we get during student teaching are sent down from Heaven. And the real kids, the ones we get after we’re hired and on our own, are sent up from the Other Place.
What I’m saying is it might be very helpful to observe these same kids whom you currently have in another teacher’s classroom.
It was around the end of the first quarter of my third year in the classroom when a colleague — a new hire — told me the vice-principal had suggested for her to observe me for a couple of periods. Afterward, she said, “Fawn, Joey is like a different kid in your room. I never knew he could sit still for 5 minutes! He’s out of control in my room. In your room, he just… blends in.”
Apparently Joey behaved differently for different teachers or at least in different settings. The teacher didn’t come back the next year — and this made me sad because she worked hard and wanted to be a teacher. There were other teachers who left the school after putting in just one year. It was a “tough” school — plagued with the usual inner city inadequacies and brokenness.
Having said that, I find kids are kids. I’ve taught in the poorest neighborhood and in the most affluent. Kids who live in fancy homes have better rides to school and wear designer labels, but at their core, they are kids who mostly want to learn and not be shunned at the lunch table.
We can’t say we possess great classroom management skills if we could pick and choose where and whom to teach. There’s a quote out there that I like: Parents are sending us their best; they’re not keeping the good ones at home. So, if we took the students out of the classroom-management-success equation, we are left with two variables: the teacher and the classroom.
The Teacher
LOVE the kids. Fine, we don’t have to love all of them because inevitably each year there’s always one (or two or three) who pushes all our buttons and makes us throw wild tantrums at home. But aren’t we supposed to be tougher than the toughest kids? How is this child’s home life? And is this child behaving like this in all her classes? There’s something to be said about killing ’em with kindness. Why are we in teaching if we didn’t love children and love helping them learn?
Show students respect. It should be the other way around — that kids must automatically respect us for our age and our college degree. But whom are we kidding. We all know of a few adults with advanced degrees who need to stay the hell away from us because they’re mean and psychotic. Kids tend to misbehave more for teachers whom they don’t respect. Do we honor their struggles and offer to help? Do we show up at their games and show genuine interest in something they do outside of school? Do we say please, thank you, and sorry each and every time that warrants it? Do we spend time outside of class to help a kid like we said we would?
Command respect. Respect has to be mutual. Like trust. We have a great opportunity to be a role model for many kids. We can’t command respect by being “pals” with the students. We all know of parents who try to be buddies with their kids. We should never ignore a disrespectful comment/tone/gesture from a student. Because if we do ignore it, it won’t be the last time it happens. How do we speak of our colleagues and administrators to students? How do we speak of our family to students? Are we consistent and honest with them? Do we follow through with consequences?
Have a sense of humor. When was the last time we laughed with the kids? The lighter moments make us more approachable and compassionate. When was the last time we shared a bonehead mistake we made? Who makes us laugh? Humor allows us more room to breathe when we need to get tough with a kid.
The Classroom
Have good lessons. I can almost tie every misbehavior or off-task behavior to the lesson itself. A good lesson is no good until it’s delivered well. Logistics. A content-rich lesson that doesn’t take into account student movement and/or material management is asking for all sorts of mayhem. Please don’t envision a “good” lesson only as a hands-on task that involves group work. A good “lecture” — aka direct instruction (maybe) — should capture students’ attention too if we drew them in with questions and invited them to make conjectures along the way. Good story telling [that relates to the math topic] will have their eyes wide open and ears perked up. Good lectures are awesome.
Establish routines:
One of the best things we have established school-wide (K-8) from the CHAMPS program is a hand signal for silence. What’s your signal? And we need to wait for that complete silence before we speak.
Kids will forget some of the routines and look at us like we’re crazy when we remind them. So, remind them and don’t look so crazy, like don’t make a big deal out of it.
We need to remember that kids [and adults] crave structure. We are creatures of habit. A good structure does not mean it has to be fixed; it means it’s flexible. Like a building that’s earthquake hearty.
Noise level. What is our tolerance level? Dead quiet has to mean dead quiet. Whispering is not dead quiet. What’s the appropriate noise level for small-group work? How often do we find ourselves yelling? There’s no rule that says we can’t stop the activity — especially when it comes to safety — if kids aren’t following protocol.
The ONE thing. Do our kids know the one thing that upsets us? My one thing is I hate mean people. So I get really, really upset when I see a kid doing something mean to another. The lesson stops. Everything stops because this is a big deal. Most of them will say that they were just playing around. We talk about that — playing and inadvertently hurting someone. Our classroom needs to be a safe place. And I want this to be a money-back guarantee with kids; it’s my one thing.
I’d taught at my first school for 8 years before I interviewed with another school. Near the end of that interview:
Vice-Principal: Fawn, there was one thing in particular that your former vice-principal had shared about you that stuck with me.
Me: Yeah?
VP: He said, She was the only teacher who could get our eighth graders to walk perfectly in a straight line and quietly from her room to the gym. How did you do that?
M: I just asked them to.
VP: You just asked them?
M: I mean they know what quiet means and what a straight line is. I told them that we needed to show respect to the other teachers and their students when we pass by their classrooms. We show this respect by walking quietly and orderly so we don’t disrupt them.
VP: How long did it take to get them to do that?
M: First time. Well, we simply didn’t move unless they were quiet and lined up. I saw pride in them as they walked. At least one teacher would happen to watch them go by and complimented them. When we got back to our room, I always thanked them and told them they made me proud.
Barbie Bungee Revisited and Better Than Yours Class Lists
This year I've taken away a lot of my step-by-step instructions for the Barbie Bungee activity that I'd posted 1.5 years ago. They get no handouts, only some verbal instructions:
[Pointing to the ceiling] See that gob of tape up there? That's leftover tape from previous years where Barbie had taken her jump. It should be at 3 meters up. Well, a small ruler will come out perpendicular (somehow) to that pole where the tape is, and that's Barbie's jumping platform. The ruler is like her diving board.
The goal is to give her the most thrilling jump — her head dips as close to the ground as possible without actually touching it. Yes, her hair hitting the ground is fine. Her jump line is made of rubber bands tied together with slip knots. (Why must we use brand new rubber bands?) You'll work in groups of three, says Instant Classroom.
So, aside from the Barbie doll, what do you think your supplies will be?
Rubber bands! How many? Lots! A hundred!
Try six. Actually seven, but one must be completely wrapped around her ankle, like this.
With only 6 rubber bands, your job is to figure out how many more rubber bands she'll need for the most thrilling jump from 3 meters.
Can we weigh her?
This is like the Vroom car!
So we have to graph, then do the extension thingy. Extrapolate. Oh, the equation is in slope-intercept form!
(We've been looking at word problems and writing linear equations that would be more appropriate in standard form or in slope-intercept form.)
Your team will have until the end of tomorrow's class time to submit your number of additional rubber bands you'd want.
For easier management of the rubber bands, I get them ready in bundles of 7, one to each group for testing and data gathering, and in bundles of 10 and extras to give out as requested on jump day.
I liked the messiness of their initial work. (I didn't give a handout or many instructions for Vroom! either, and they did fine.) Kids doing whatever they think they should do, measuring incorrectly, plotting ill-looking graphs, talking and criticizing one another. I was debating when I should intervene, but it was good for me to just observe and listen in.I waited until the next day to point out stuff. Actually I never told them what they should do, I tried instead to ask them how something should be done. I don't think one single idea came from me — someone always had the answer I was hoping for, so all the "correct" ways to do things came from them. My phone apparently didn't have enough memory after this one clip. It was fun. (One kid also brought up that this was like the Stacking Cups lesson that we did.)
This might seem to you a DUH! share, but I only thought of it earlier this year, and I feel like I invented the paper clip. We all have class lists, of course. But is each of your class lists on a strip of paper like this? And in different colors? I didn't think so.
I have semi-thick stacks of these to use for just about everything. What a pain to write down kids' names for this and that. Instead I just pull out a strip and highlight so-and-so's name and note the reason.
I staple one set of strips together, put a date on it, and kids pass it around to each other to sign in for after-school help — they just need to put their initials next to their names.
Those who need to come in at lunch recess get their names highlighted on the strip.
I use it as a hall pass when I need to send 2 to 3 students at a time to the library.
I highlight a kid's name whose parent I need to contact, then use the back of the strip to make notes from our conversation.
It's a great tally sheet for whatever during class.
Endless uses.