A Book: Thinking Mathematically

I'm re-reading Thinking Mathematically, an assigned book from a math course I took years ago in Portland. I was teaching science at the time but signed up anyway because I've always loved math.

Thinking is still so good and resonates much more now that I've been teaching mathematics.

In the Introduction, under "How to use this book effectively!":

Recalcitrant questions which resist resolution should not be permitted to produce disappointment. A great deal more can be learned from an unsuccessful attempt than from a question which is quickly resolved, provided you think about it earnestly, make use of techniques suggested in the book, and reflect on what you have done. Answers are irrelevant to the main purpose of this book. The important thing is to experience the process being discussed.

... our approach rests on five important assumptions:

You can think mathematically Mathematical thinking can be improved by practice and reflection Mathematical thinking is provoked by contradiction, tension and surprise Mathematical thinking is supported by an atmosphere of questioning, challenging and reflecting Mathematical thinking helps in understanding yourself and the world

These assumptions need to live in our classrooms.

The problems in Thinking are mostly brief and simply stated -- yet each one has the potential to make you linger a bit longer because you want to savor your own thinking. Not even productive struggle, this is sweet struggle.

How many rectangles are there on a chessboard? [Page 43]I have just run out of envelopes. How should I make myself one? [Page 35]A certain village in Jacobean times had all the valuables locked in a chest in the church. The chest had a number of locks on it, each with its own individual and distinct key. The aim of the village was to ensure that any three people in the village would amongst them have enough keys to open the chest, but no two people would be able to. How many locks are required, and how many keys? [Page 176]

I'm finding out that the 2nd Edition came out in 2010. Amazon does not have it in stock currently, but when it does become available, we can rent it for $54.77 or buy it new for $91.29. What??

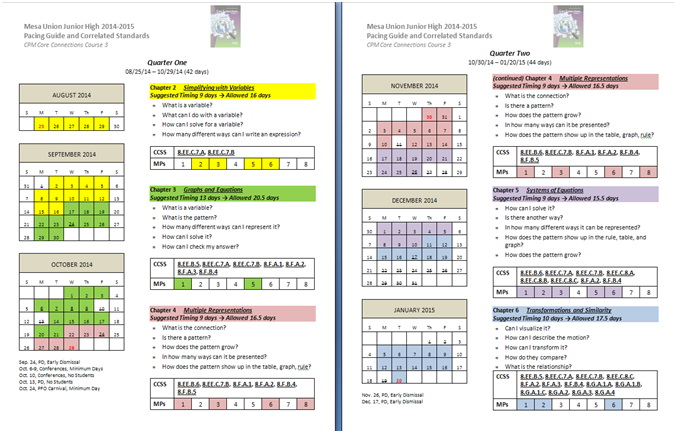
Pacing Guide

For someone who has openly admitted to not following curriculum pacing guides, I sure spent a ridiculous amount of time churning one out. Our middle school is adopting CPM Core Connections 1, 2, and 3. Aside from our own reviews, the decision to go with CPM were also based on:

1. Desmos is embedded in many lessons

2. Other teachers' reviews, including Riley Lark's

I don't know if this would be of any use to you, but I might as well share the doc math 8 pacing 2014-2015. It's kinda pretty.

I replicate our school calendar and put in all the holidays and half-days. I go to each chapter in CPM and write down the guiding questions. Matching up the standards to each chapter was a pain in the ass. (CPM does it the other way around: they have the standards in one column and the different lessons that cover those standards in another.) The suggested number of days for each chapter does not include assessments, so I add about 6 days on top of whatever CPM recommended. I'm going to post the pacing guide near my desk — probably the only document I will print in full color this school year.

(Oh, I took out Chapter 1 because it's on problem solving. C'mon, I got this.)

Then I'm going supplement it like crazy. I can't teach straight from the textbook. Just can't. So the 6 days that I add to each chapter will hopefully allow us some wiggle room to do other stuff.

Other stuff includes, but not limited to, what you see on the right sidebar of my blog.

We also need time to begin each class period with math talks because it was one of the most powerful things we did last year. (Grrrrr. Just realized that most of the images on the math talks site are not there. Why now.)

I was brainstorming with a couple of 6th grade math teachers at another district, and we were listing out a possible warm-up/math talk schedule, something like:

• Monday: number talk (spreadsheet that you can take from and add to)

• Tuesday: visual pattern

• Wednesday: estimation 180

• Thursday: fun fact, or WYR, or Keeping Skills Sharp, or SBAC/review question

• Friday: personal reflection

My assignment this year looks almost like last year's: 2 sections of Math 6, 1 section of Math 8, and 1 section of Geometry [1].

I wish you a healthy school year. Teach what you love and love the kids. Follow the rules, but break a few if doing so makes it better for the kids. 

[1] I'm happy to say that we will no longer be tracking kids in math. However, we need to finish out what we'd started with these 8th graders who took Algebra last year as 7th graders. So this group will do some geometry, some stats, and a whole lot of problem solving.

Playing With Math: Stories From Math Circles, Homeschoolers, and Passionate Teachers

Sue VanHattum's blog Math Mama Writes was one of the earliest math blogs I frequented. Sue and Shireen, Dan, Kate, and Sam were among the first people who showed me — through their passionate writing — that there was an online community where we may share the teaching and learning of mathematics in the classroom.

When I started this blog, Sue dropped in to leave a comment or two. Or five. We even talked on the phone, and she shared with me how her son got his name. I remember reading someone's post and wanting to leave a comment because I connected with the piece and its author in some small way, and inevitably I would find that Sue had already left a comment. This happened over and over again. I felt we were reading the same stuff; and the same stuff touched us in similar ways.

Then, within a year Sue asked if I would like one of my posts to appear in a book she was putting together.

So, it's with much love and honor that I get to help launch Sue's new book:

Playing With Math: Stories from Math Circles, Homeschoolers, and Passionate Teachers brings together the stories of over thirty authors who share their math enthusiasm with their communities, families, or students. After every chapter is a puzzle, game, or activity to get you and your kids playing with math too.

To know Sue is to know that she loves teaching and learning mathematics, and she loves writing, therefore this book — this work of consummate love - has to happen.

Playing With Math is really a collection of love stories because the authors, including yours truly, want to share something we're pretty crazy about. It's the stuff we do beyond the regular school day — we play with math after hours, at the dinner table, on a napkin at the coffee shop, with our own child or with a neighbor's child, at a family picnic, with our in-laws whom we don't even like.

So, today is the first day of our crowd-funding campaign to cover production costs. We're hoping to find support in our community of teachers and parents and math connoisseurs — a community of people whom I adore and respect. You can contribute anything from $1 to $1 billion. But for a contribution of $25, this wonderful book will be sent to you as soon as it's printed. Please see more details here.

Thank you so very much.

Have You Had Your Math Munch Today?

I read Math Munch's latest post yesterday.

Today my students sketched out their birthdays inspired by the post. Just as Brandon Todd Wilson limited himself to only one hour to work on each day's number, I gave my students exactly one class period to do theirs. The perfect kind of year-end lesson that does wonders for Eighth Grade-itus. Thank you to Anna, Justin, and Paul for the beautiful and passionate work that you share on Math Munch!

Math Taboo Game

Pretty sure I lied to my algebra students when I announced abruptly, "Let's play this game. It's a lot of fun!"

I had never played the game before as I just thought of it when I spoke the words. (They were doing polynomial factoring and were being so nice and quiet. I can't stand "nice and quiet" in my math class, so I had to disrupt them and wanted to play a game.)

I thought of playing Taboo using math vocabulary. In case you're not familiar with it, the object of the game is get your teammate to say a certain word on the card without using the "taboo" words. For example, describe VEAL without using the words CALF, YOUNG, PARMIGIANA, CUTLET, BOX.

My instructions:

1. Take out a piece of paper, fold it into quarters and tear neatly so you have 4 small pieces of paper.

2. Open up your math book and look for vocabulary words that we've covered already.

3. At the top of each piece of paper, write a word (or phrase) that you want people to describe, box this word up.

4. Then underneath this word, write 4 taboo words that the describer may not use.

5. Don't share what you're doing, wouldn't be fun if others saw what you wrote.

6. I need each person to complete 4 of these.

7. When done, fold each piece of paper exactly in half — like this (you have to show them) — and drop them all in this bucket.

A few simple rules:

1. Class is divided into 2 teams, Team X and Team Y.

2. Team X goes first: one person from Team X comes up to front, reaches into bucket to get one slip of paper and has 60 seconds to describe as many words as possible to the teammates.

3. Teacher stands next to the describer to make sure none of the taboo words or the main word itself is said — round is over if this happens.

4. Skipping a word is not allowed.

5. Hand gestures are okay (one describer today put his arms out in parallel fashion to get his teammates to say "perpendicular" — who's his math teacher?

6. Teacher reserves the right to help out whichever team she likes better.

So, yeah, we had a ton of fun. It was the last period of the day, and they didn't want to leave when the bell rang!

This went so well that I'll make a "real" game out of this. I'll print the words onto mailing labels. Then I can stick them on the back of playing cards so they are shuffle-able.

Lego Pieces and Feasible Region

This afternoon I came across a tall stack of Lego® boxes stored in my son's closet; they reminded me of a lesson.

(My son just turned 20 three days ago. I can still see him playing with his Lego sets for hours on end when he was younger. And when he ran out of floor space in his room, he took over our entire living room, and we were happy to let him.)

Simple lesson — (I was looking around and this site cited the Mathematics Teacher, Vol. 92, No. 2 February 1999 titled "Promote Systems of Linear Inequalities with Real-World Problems" as source for this problem.)

You own Funky Furniture, a store that makes tables and chairs out of Lego pieces to sell. A chair is made of one large and two small pieces. A table is made of two large and two small pieces.

Currently you have 8 small Lego pieces and 6 large ones. If the profit for a table is $16 and for a chair is $10, then how many tables and chairs should you make to maximize profit for your Funky Furniture business?

I know there are a ton of "systems of inequalities" problems out there, but playing with Lego pieces is just more fun. Students can see the "tables" and "chairs" as they build them. I have the kids work in pairs, so each pair gets a Ziploc bag of 8 small pieces and 6 large pieces. Nobody leaves my room until all the pieces are back into the baggies and returned.

If you don't have Lego, maybe the kids can bring theirs in? There's ebay. Or you can just use centimeter cubes and inch cubes, or dice and cubes, or Cuisenaire rods and round counters. Or you can just print small and large rectangles on construction paper for them to cut out — flat models are better than no models. Anything they can manipulate is fine.

We'll be ready for this lesson next week as we just wrapped up systems of linear equations right before break.

And I just remember this fun problem about Mrs. Murphy's Missing Laundry. Have you seen it? Here's the handout. There's no manipulative involved, but kids like to solve mysteries too.

Mailing Labels Make Retakes Less Crazy

Some years ago I was either talked into or gently coerced into teaching three classes that I had zero qualifications for.

I was asked to teach French because I could count from one to twenty in French, plus I could sing Frere Jacques. Or was it because I'd told someone that my favorite sing-along song while growing up in Vietnam was Dominique by Soeur Sourire. Thank God that was only a quarterly elective class. Oui.

Then I was hired to teach AP Chemistry. But I have to tell you about that job interview.

Principal: Fawn, sorry to keep you waiting [for 45 minutes]. I know you're here for the math position. But we already hired someone for that.

Me: You're fucking kidding.

P: We really need someone to teach AP Chemistry.

M: What's that?

P: You have all these science classes on your transcript.

M: My degree is in Biology. But I took a lot of math too.

P: Yes, right. So you can certainly teach AP Chemistry.

M: I want to teach math. When did you give away the math position?

P: To the guy who just interviewed. He walked right past you.

M: That asshole?

P: You'll be perfect for the chemistry position.

M: Okay, fine. That's it? I'm hired?

P: Yes! Welcome to our school!

Maybe I lied about what I'd said in that interview. But I swear to God the rest of it is true. I wonder how many current teachers were hired the same way I was — we looked good on paper, even if nothing matched up. I worked so hard that year just to be a couple pages ahead of the students, but clearly these bright kids deserved better.

I also taught writing. A colleague volunteered me for this elective. I did know the secret to writing, however, so I enthusiastically accepted the challenge. The secret to writing, as writers will tell you, is to write! Just write, they say, keep that hand moving.

Where am I going with this post? Right. Mailing labels. Mailing labels.

But back to writing. When I taught writing and didn't know how, I just gave the students daily writing prompts. I didn't even read what they wrote unless they requested me to. But I ALWAYS wrote along with them. Their prompts were my prompts. Like shameless addicts, we wrote ferociously and freely. We liked our "shitty first drafts" and rarely edited our work. We'd stop 10 to 15 minutes before class ended to share snippets of our writing aloud, if we wanted to.

Yes, the mailing labels! I had an idea to print out a full sheet of mailing labels with writing prompts on them!! When kids walked into class, they peeled off a sticker, slapped it on their journal page, and started writing. I still have a copy of the 7 pages of stickers — at 30 a page, that's 210 prompts! I lifted most of these prompts from one of Natalie Goldberg's books.

Then I used the same idea when I started teaching math because I wanted kids to write in math too! So I took a lot of math writing prompts from this book. I was doing this daily which became costly, so we cut it down to just weekly writing. I hate the wasted time for kids to copy down the prompt or the question; placing the prepared sticker on their journal page saved this time.

But, this year I found the best use for the mailing labels with Standards Based Grading (SBG). School is in full swing now and there are a lot of kids coming in at lunch time for retakes. Currently, and because this is our first year with SBG, we can only manage to assign selected questions from the textbook for reassessments. I either have to tell them what the problems are when they come in or give them a piece of paper that has the problems on it, then they have to copy all this information on notebook paper: section title, page number(s), and which exercises. Without this information, I can't correct their papers.

Now, when a kid comes in, I just ask what he/she wants to reassess on and slap the mailing label with all the info on their paper! (They have to sign up online first to let me know.) The number of labels I make is equal to the number of scores of 1s and 2s earned for that assessment.

I think it's brilliant. Imagine the endless possibilities.

Why Wait for Calculus?

(I just had my very best lesson yesterday, on a Friday, thank you. I feel almost brilliant right now. And I only feel like this once every 47 years, so please stay and read this post!)

My own kids tell me they will stock up their dorm rooms and apartments with junk food and soda when they move out to make up for these years of deprivation. (And this is supposed to make me feel bad.) So when I intentionally bring home a snack, like this bag of kettle corn, I usually find it empty within 24 hours. But seeing the empty bag made me think of a volume activity that I could do with my 6th graders with all these other bags of Orville Redenbacher's popcorn.

But the activity I had in mind — maximizing the volume of a box — is commonly done in a pre-calc or calculus class. These are my 6th grade babies. But didn't we do okay with approximating the volume of a torus via my doughnut lesson? So, why can't we do this too? I have to get rid of the popcorn.

I randomly assigned the kids in pairs, gave each pair two sheets of white copy paper. I told them to use one paper at a time to make a box — the goal is to make the box as big as possible so it'll hold the most popcorn. But the box must be made simply like this: cut off 4 corners from the the paper, then fold up the sides and tape them together. I used a half-sheet (so they couldn't duplicate mine) to demonstrate what I meant.

They quickly went to work. A few students were NOT cutting off square corners, so the top edges of the sides didn't line up. Two groups folded in their papers, in addition to cutting off corners, so they had to re-do.

Ryan and Annamaria wanted to make a shallow box. Ryan said, "... it doesn't matter how high it is."

Rapha and Cristian made the biggest corner cuts that I saw in the first round.

Mike's and Roy's first box was the shallowest in the class, but they changed their mind for their second box.

With 10 minutes left in our first hour together, I asked the kids to measure the box and find the volume. They had no trouble with this since we did the doughnut. They recorded the volume inside each box, and I tacked them on the board. (The butter seeped through in few of the boxes.)

Well, that was fun. I pointed out that two of the bigger boxes were over 1,000 cubic centimeters. The bell rang. I said, "We'll wrap up this afternoon."

I didn't know what I was going to do to "wrap up" the lesson. The microwave actually overheated — my room stank of greasy popcorn.

There was a confidence in me, however, that the kids would help me figure out how/where this lesson could go next. I began the afternoon hour by going over what they'd learned in the morning. They said:

The four corners must be of the same size. (I never told them this in my instruction.)

Each corner must be a square. (I didn't tell them this either. Not everyone was convinced of this, so I cut non-square corners to show them.)

There was a limit to how big the square could be. (I loved this! And this made me ask, "Is there a minimum to the size of the square?" Their eyes squinted, almost as if they were trying to "see" how small these corner squares could get. One kid said, "No. Technically, no.")

The volume numbers that people wrote down could be wrong.

By then they understood the different boxes and their volumes depended on the size of the corner squares that would get cut off. We focused on this. I asked them to draw a 10 x 12 rectangle in their math journal. We removed 1 x 1 corners from this rectangle and found the volume. I guided them through the next 2 x 2 corners. They continued on their own.

Then I gave each kid another white piece of copy paper. We measured the length and width of the paper and agreed that the paper was 28 cm x 21.5 cm. I asked them to build a systematic table like the one they just did in their journal. I said something like this, "Because you now know how to figure out the volume without actually cutting and making the boxes, see if you could figure out what size square the corners should be to maximize volume."

I saw kids high-fiving each other, "The corner has to be 4 by 4!" Rapha and Cristian beamed after congratulating each other, "That was one of the boxes we made!" We ended class with that. I swore I felt myself tearing up.

On Monday we'll play around with this applet.

And we'll ask Wolfram Alpha to take the first derivative of the volume for us. (I'm pretty sure the class could write this equation V = (28-2x)(21.5-2x)(x) for me to enter into WA.) Well, I actually just did it, and WA gives the side of our corner square as approximately 4.01965. My kids got 4 — pretty damn good for 6th grade brute-force math. Now that I'm writing this, however, I am really most proud of how well the kids had worked together. I randomly paired them up — a handful of the pairs were like the odd couples: high/low, shy/outgoing, squirrelly/quiet, jock/nerd, princess/cowboy. There was not a whisper of whine when their names were called to pair up. How did I get so lucky?