Number Talks for Middle Schoolers

If I may recommend only one thing to K-12 classrooms, that thing has to be number talks. As a warm-up routine, it usually takes less than 10 minutes, yet it pays dividends in building number sense, connecting computational strategies, honoring flexible thinking.

I finally finished this set of 90 number talks for middle schoolers — enough for a full school year if you do this routine every other day. But I hope you’ll also share it with your elementary and high school teachers to inspire them to create their own or modify these.

I’ve already shared my notes about the routine on the first 4 slides. I just want to add that each slide — or each group of 3 slides — is intentional, meaning there are several strategies that I hope your students and you will explore and share. My goal is also to tweet these out, one at a time, and include people’s responses on a slide following it.

You know what you should do on the other 90 days. :)

Changing Up Popular Warm-Up Routines

As with any task, whether it's a warm-up or a curricular task, I try to think of ways to get more student engagement, tap a different thinking modality, and just to change things up.

WODB has become a common acronym in classrooms for good reasons. (Actually, does it qualify as an acronym like NATO since I've never heard it pronounced as a word? Y'all are still saying Which One Doesn't Belong, right?) Take this first one I see on the site. (I added the numbers 1-4.)

I do my homework first, in the order that the shapes come to my brain:

• #2 doesn't belong because it's the only non-triangle.

• #4 doesn't belong because it's the only shaded shape.

• #1 doesn't belong because it's the only one with exactly one line of symmetry.

• #3 doesn't belong because it's the only obtuse triangle.

With students, I ask them to give me a blank grid and get ready to draw in each box as they listen to my clues. I tell them to make quick sketches as they may need to make changes when they hear new clues. I can give them the clues in any order I choose. But, we'll stick with the order above.

• #2 doesn't belong because it's the only non-triangle

A possible sketch:

• #4 doesn't belong because it's the only shaded shape.

• #1 doesn't belong because it's the only one with exactly one line of symmetry.

• #3 doesn't belong because it's the only obtuse triangle.

Students can then share their sketches and critique each other's work. The reveal is fantastically fun.

Estimation 180 is another popular one. From the site's Day 6:

Instead of asking students for an estimation, I ask:

One of the four numbers below is the correct number of almonds in the 1/4 cup pictured. Which one is it and why did you choose it? Which number do you believe is way off?

8

15

28

40

I find their reasoning and conversations are tighter this way — more focused. I'm also one of those people who dread having to guess at something, even with a visual clue. With younger students, I'd give them 3 choices instead of 4.

Open Middle is another well-loved routine. This one is filed under Grade 4, Equivalent Fractions. (I added the digits A-G.)

Directions: Use the digits 1 to 9, at most one time each, to make three equivalent fractions.

I mark the digits 1 through 9 on red/yellow counters and put them into a baggie. I reach into the bag and randomly pull one out. Say, I pull out a 5. I call on a student [randomly] and ask, "Which space (A-G) can the number 5 not be in?"

There is a big difference between asking the above question versus, "Where do you think the number 5 goes?" The chance of answering this question correctly, if 5 is used at all, is 1 out of 7. The former question is much safer to tackle. This routine engages the whole class on one number at a time — we get deeper thinking when we can focus on one thing and while building on each other's thinking. And I very much love it when students are given opportunities to honor and build on their classmates' reasoning. After a few suggestions, a student might conclude that the number 5 can go into the discard pile. (It's also common for students to use a number more than once or use a number not allowed, so the counters alleviate this mistake.)

Also, I need to share that this problem was posed in an online workshop I attended yesterday, and I didn't even attempt it because these are my constant truths:

1. Someone else will come up with the answer before I do.

2. The answer will be revealed before I get to solve it, so no point in me ever working on it.

How many of our students also hold these truths? I understand this was a workshop for teachers and time is limited and sharing is good and all that. I'm just thinking about best practices with students though. 

[Added 10/17/2020]

I meant to include a numerical WODB example because there are a lot of possible solutions, it's always fun to see what the kids come up with. Here's the first numerical one on the site. (I added the letters A-D.)

I work on it first.

• D is the only non-square number.

• A is the only single-digit number.

• C is the only number divisible by 5.

• B is the only even number.

I give the students the above clues in the same order. However, students may not erase a number as they revise, they may only cross it off so that I may see what they had originally.

Good-Enough-for-Now Curriculum

I did my first webinar last week as a precursor to my talk at NCTM's Innov8 Conference next month. I thought it went okay — or horribly — just tough to be the only person with the mic and not being able to actually see the attendees. It was weird.

There are a few slides from the webinar that I'd like to share here mainly because I'm still thinking about them and writing anything down helps me set the wobbly gelatin.

Two weeks ago I presented at an independent school that's Preschool through Grade 8. Afterward, I was given a quick tour of the school — the 33-acre campus gleamed with pride in its thoughtful architecture, manicured grounds, state-of-the-art this and that, and a smorgasbord of elective offerings, including Mandarin and photography.

My school is Kindergarten through Grade 8, and the similarity between my school and this independent school pretty much ends there. I teach four classes, my smallest class has 23 8th graders, the other three, all 6th graders, have 32, 35, and 36 students. We're a Title 1 public school.

I bring up the private school and my public school because, like apples and pomegranates, they are quite different. So, when we do PD and share whatever it is that we share about education and serving children, we need to be mindful about the space that each teacher occupies in her building and be mindful about the children who come into that space.

When someone shares something with me, one or more of these thoughts cross my mind: 1) I can see how that would work with my students, 2) I can see how I might adapt this to fit my kids, 3) This person is afraid of children or unaware that children are people, 4) Nobody cares.

Likewise, when I have the stage to share, I'm assuming you have similar thoughts of my work. But I beg you to think about the space that I share with my students.

Below is a quasi rating scale of "critical thinking demand" that I'd created to place the types of tasks that I regularly give to my students. And this scale is only possible because I'm mindful of the tasks' contents and my own pedagogical content knowledge to facilitate these tasks.

What are these six things? The resources for these are on this spreadsheet.

1 & 2.  Assessment and Textbook: We're using CPM. [04/07/2022: We now use Open Up Resources and Desmos.]

3.  Warm-up: Due to our new block schedule, we've only been doing number talks and visual patterns. 

4.  Problem-of-the-Week

5.  Task

6. PS (Problem Solving)

Do these 6 things align to the curriculum?

The slide below shows the 4 types of tasks that are aligned to the curriculum, or that when I pick a PoW or Task, I make sure it correlates to the concepts and skills that we're working on in the textbook. Therefore, it's entirely intentional that the warm-up and PS are not aligned because critical thinking and creative thinking are not objects that we can place in a box or things that I can string along some prescribed continuum.

All 6 types of tasks are of course important to me. I try to implement them consistently with equal commitment and rigor to support and foster the 8 math practices.

Which ones get graded?

I don't grade textbook exercises, i.e., homework, because I can't think of a bigger waste of my time. I post the answers [in Google Classroom] the day after I assign them. I don't grade PS because that's when I ask students to take a risk, persevere, appreciate the struggle. I don't grade warm-up because I don't like cats.

I'm finally comfortable with this, something I've been fine-tuning each year (more like each grading period) for the last 5 years. I could be a passive aggressive perfectionist — or just an asshole when it comes to getting something right — so it's no small admission to say that I'm comfortable with anything.

It's about finding a balance, an ongoing juggling act between building concepts and practicing skills, between problem-posing and answer-getting, between teacher talk and student talk, between group work and individual work, between shredding the evidence and preserving it. Then ice cream wins everything.

Here's the thing. We want to build a math curriculum that makes kids look forward to coming to class everyday. I trust that that's true for more than half of my students — this could mean anywhere between 51% and 80%. I think we're doing something wrong when kids look forward to just Measurement Monday, Tetrahedron Tuesday, or Function Friday. Math should not be fun only when students get to play math "games"!

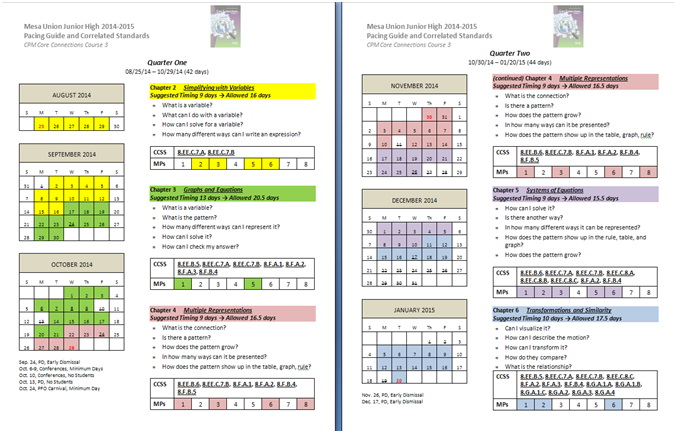
Pacing Guide

For someone who has openly admitted to not following curriculum pacing guides, I sure spent a ridiculous amount of time churning one out. Our middle school is adopting CPM Core Connections 1, 2, and 3. Aside from our own reviews, the decision to go with CPM were also based on:

1. Desmos is embedded in many lessons

2. Other teachers' reviews, including Riley Lark's

I don't know if this would be of any use to you, but I might as well share the doc math 8 pacing 2014-2015. It's kinda pretty.

I replicate our school calendar and put in all the holidays and half-days. I go to each chapter in CPM and write down the guiding questions. Matching up the standards to each chapter was a pain in the ass. (CPM does it the other way around: they have the standards in one column and the different lessons that cover those standards in another.) The suggested number of days for each chapter does not include assessments, so I add about 6 days on top of whatever CPM recommended. I'm going to post the pacing guide near my desk — probably the only document I will print in full color this school year.

(Oh, I took out Chapter 1 because it's on problem solving. C'mon, I got this.)

Then I'm going supplement it like crazy. I can't teach straight from the textbook. Just can't. So the 6 days that I add to each chapter will hopefully allow us some wiggle room to do other stuff.

Other stuff includes, but not limited to, what you see on the right sidebar of my blog.

We also need time to begin each class period with math talks because it was one of the most powerful things we did last year. (Grrrrr. Just realized that most of the images on the math talks site are not there. Why now.)

I was brainstorming with a couple of 6th grade math teachers at another district, and we were listing out a possible warm-up/math talk schedule, something like:

• Monday: number talk (spreadsheet that you can take from and add to)

• Tuesday: visual pattern

• Wednesday: estimation 180

• Thursday: fun fact, or WYR, or Keeping Skills Sharp, or SBAC/review question

• Friday: personal reflection

My assignment this year looks almost like last year's: 2 sections of Math 6, 1 section of Math 8, and 1 section of Geometry [1].

I wish you a healthy school year. Teach what you love and love the kids. Follow the rules, but break a few if doing so makes it better for the kids. 

[1] I'm happy to say that we will no longer be tracking kids in math. However, we need to finish out what we'd started with these 8th graders who took Algebra last year as 7th graders. So this group will do some geometry, some stats, and a whole lot of problem solving.