My Other Math Sites
Lessons From the Classroom
What Our Students Thought of Ability Grouping
A month ago I wrote about Ability Grouping and within that week asked my students for their thoughts, but I've been swamped with work to share any sooner. My teaching assignment was:
Geometry: 32 students. All were 8th graders, largest group we'd ever had, 45% more than the year before. A handful of them were not ready for the rigors of this high-school equivalent course; it was more due to scheduling that they ended up here. (We have about 200 kids in the junior high, 2 sections of each level, so it's not easy to be flexible with our schedule.)
Algebra 1: 38 students. Ten were 7th graders, the rest were 8th graders, and 3 of the 8th graders worked independently out of the Algebra Readiness textbook at midyear as they struggled to continue on, but they joined the rest of the class for all problem-solving group tasks which happened about twice a week.
Math 6: 69 students in two classes, but 2 of them were in RSP for math, so I only saw them on Fridays.
My colleague Erin helped me give her students the same survey questions. I don't know her exact roster counts, but she taught two classes of Pre-Algebra (all were 7th graders) and one class of Algebra Readiness to 8th graders. I began by telling the kids a little bit about ability grouping at our school, that it existed in grade 7 and grade 8, that we tried to place them based on several measures (grades, work habits, CST scores, benchmark tests). I did not tell them how I felt previously or presently about ability grouping, but I wanted to learn what they'd thought. I gave each student this strip of paper and asked them to check one box.I then added these three questions: I'm going to summarize what boxes the kids checked for both parts above like this:
From the Geometry kids (there was some city-level academic competition going on that day, so more absences than usual):
From the Algebra 1 kids:
From the Algebra Readiness kids:
From the Pre-Algebra kids:
From the 6th Graders:
So, about 83% (151/183) of the kids said YES to ability grouping. Sure, there were a few kids who seemed to have conflicting responses by checking NO to the first question but had more YES responses with the 3 questions that followed, and vice versa. I don't know. I do know this: Erin and I really love our students and love what we do. She has a math degree, I do not, but we both love problem solving and are proud math enthusiasts. She let me talk her into going for a week-long training in Palo Alto to jump start — actually it was more of a revival of — a Math Teachers' Circle in our area. I want to believe that Erin and I made a difference in how our students felt about their learning of mathematics. Doug left a comment on my Ability Grouping post. His last paragraph strikes the perfect note of what I want to say right now:
I wonder if the bigger problem is teaching students to not be so concerned with who is "ahead" of whom. Maybe the problem has less to do with what class you put the students in, and more to do with how you treat them once they get there. We need to foster the growth mindset. Maybe our fixed-ability mindset (ala Carol Dweck etc.) primes us to be unnaturally sensitive about placement. Most of us will live and die always knowing there is someone, lots of people, more competent/talented/accomplished than we are at everything we do. But we have to live for ourselves, and pursue what we care about, regardless. The bigger issue is making sure every classroom has a good teacher presenting quality material. Then it doesn't matter who is in what room with whom.
Thank you, Doug.
Discount and Sales Tax
Instead of doing the above exercises in our textbook, I had my 6th graders do this:
Shopping Contest at Target
Let’s pretend Target has a contest. The contest is for shoppers to find merchandise from their online store.
Contest rules
You must choose at least 10 different items.
The items must come from at least 5 different departments, such as footwear, kitchen, clothing, toys, etc.
You may buy more than 1 same item — you can buy 2 or more packs of athletic socks.
The winner
The winner is the shopper whose merchandise receipt totals exactly $500 or closest to it — without going over. They get to keep all the merchandise!
Your task
Phase 1: Go to Target’s website and find some items that you’ll want to get for yourself and your family. List the items and their original prices in the table provided separately.
Phase 2: The store manager (really, it’s your teacher) then announces the percent discounts for different categories of merchandise. Write these down below. Apply these discounts to your items and calculate the new sale prices.
Phase 3: The current tax rate for our city is 8%, so you must add this to your total. In this phase, you get to add or remove items on your shopping list to reach the target goal of $500 without going over.
I only did Phase 1 with the kids, my sub supposedly did Phases 2 and 3 with them. (I wanted to do the rest of the lesson with them when I return from NCTM — and after spring break — but they voted to continue the lesson with the sub as they were really into the task. I'll find out tomorrow when class resumes.)
Why this task
You can change everything about it. Shop somewhere else instead of Target.
Change the rules depending on time available and access to computers. Are there enough computers for each kid or do they need to work in groups of 2 or 3? My kids were in pairs. (We have enough laptops, but at least 30% of them have issues.)
I like the idea of not going over a certain amount — $500 in this case — instead of "whoever is closest to the target price" because I think it keeps the kids more reserved in their shopping spree. Students understand that if there were only 2 contestants, then the one with a final receipt total of $154 would win over the other with a $501 total.
Kids don't know what the exact discounts are until Phase 2, so this makes it a fun temporary secret. But they know to go over budget in Phase 1 because there will be discounts — not all departments have to have discounts either. Their totals in Phase 1 were in the $600 to $700 range because they also know that it'd be easier (faster) to remove items than add them later in Phase 3. They were also told that the discounts would be somewhat realistic, meaning Electronics will get a smaller percent discount, if any, than Clothing.
They'll become more aware of how much things cost — and how quickly they add up in the shopping cart.
Handout Discount and Sales Tax
Prices, Proportions, Percents
I was in Garden Grove with my son on Sunday, and he insisted that I try this smoothie place called Tastea. With Jamba Juice and Blenders and all the other juice bars around town, I was skeptical that this joint's concoctions would be anything different. He ordered a taro milk tea and I got a Thai tea, both with boba. Just one sip and I said to him, "Let's order another round! It's a long drive home!" Soooo delicious.
While there I saw a math lesson brewing, so I picked up their menu with prices. This is the lesson with my two classes of 6th graders.
Me: (I tell them about Tastea and how I wish it were closer.) Okay, let's start with something you might be more familiar with, Starbucks. I love that now I live within walking distance from one! Do you know the different sizes that they have there?
Class: (When I refer to "class," I don't mean the whole class, of course, but somebody in the class joins in on the conversation.) Tall, grande, venti.
M: Do you know exactly how much liquid each size holds? (They make various guesses. I bring out the 3 sizes that I got from Starbucks so they have a visual.) I normally order a tall mocha frappuccino, let's say the price is $3.50. Do you think the venti, which is twice the volume of the tall, would cost twice as much, or $7.00?
C: No.
M: Why not?
C: You normally get a better deal with a bigger size.
M: What do you mean by a "better deal"?
C: (All their answers show me that they understand the idea of more bang for your buck. Then finally someone says...) Lowest unit price!
M: Right! That's why so many families go to Costco. Buying in bulk normally saves us money because the item has the best unit price. Well, we're talking about Starbucks now, so buying more is a better deal, but drinking more is not so good for our body. Let's fill in this sheet. (I pass out this handout. And here’s the key.) How do we calculate unit price? What place value should we round it to? How do you write thirty-one-cents-per-ounce?
M: Tastea has three sizes: mini, gigantic, and even more. Their teas can also be purchased by the "partea jug," which holds a gallon. I've given you the prices of the 10-ounce minis for the three different types of drinks, your job is to figure out the prices of the other sizes. You'll work in small groups to figure out these out. So, do you think the gigantic will cost twice as much as the mini because it holds twice as much?
C: No. It'll cost less.
M: How much less? Well, that's your group's job to come up with the best estimate. We have Starbucks' prices for their three sizes, you could look at how they price their drinks. But here's the sweet deal for you. You and your group mates do the math that you need to, then write down your first estimation right here in this column. Bring your paper up to me (only the "captain's" paper), give me a few seconds to figure out the percentage that your estimation is off by, and I'll write it in this column and give you back your paper. What percent do you want to see, large or small? What if your estimation were the actual price — what percent would I write there?
I tell them that they could figure out the actual price of the drink if they knew how I calculated the percentage of error. So, work work work. Think think think. What makes sense? Oh, I remind them that the percentage does not indicate if their estimation is too high or too low. So, again, what makes sense?
We also note that prices generally end in a 0, 5, or 9. So, even if the calculation tells them the price should be $4.23, they might want to change that to $4.25 or $4.20.
When they bring up their paper again with the second estimation, all I do is write their estimation again in pen and circle it — this is so they can't change their answer and I know that I've seen it. I do NOT fill in the "Actual Price" column at this time because the groups are working at different rates, and in a crowded room, it's easy for kids to see each other's papers, even inadvertently, and the game of estimation is over if they saw the actual price beforehand.
They simply move on to the next size to make a first estimation again. We repeat the process.
When all groups are done with estimations for the first type of juice — smoothies — I tell them what the actual prices are.
Now, it's their turn to figure out the percentage of error. I give the groups about 10 minutes to do so without help from me. At the end of the 10 minutes, either there's at least one group that knows how to do so and can show it to the class, or no group knows how, then I'll walk them through the calculation by asking them questions to figure this out.
They continue in the same manner for the Slushy Freeze and Specialteas on page 2. This time hopefully they'll be able to work backward from the error percentage that I give them after their first estimation.
Reasons I'm proud of this lesson:
It's about proportions, but many priced items in real life are not directly proportional. The kids knew this coming in because they've been consumers.
We get to talk about business strategies that entice people to buy the larger sizes while still make a profit. (Starbucks calls it "tall" because it rhymes with "small," but clearly the word tall naturally elongates the imagination.)
Students get to make estimations throughout, but they know these aren't "wild-ass guesses." They start with the calculation of proportions and adjust the prices accordingly. They get to critique and argue with their group mates to come up with the best estimations.
I get kids to think about percentage in a context that they can wrap their heads around. And they want to know how because their second estimation could be dead on if they knew.
It's fun that the error percentage does not indicate if their estimation is too high or too low. A few groups do go farther in the wrong direction. Oh, well — good to learn that now.
It'd be fun for me to get Starbucks or Jamba Juice for the group with the lowest total in percentage errors.
Updated 04/05/14
I got some thoughtful reflections on this lesson, I'll just share two:
I learned how to work backwards with percentages and try to get the number spot on. I also learned how business would price things by dropping the price by the perfect amount. My number sense got a lot better from all the multiplying, dividing, and reasoning. It was very difficult, which I'm very happy about. The teamwork was probably the hardest part of the project. M and I are very competitive, and we got different answers a lot. I learned how to work together with others a lot better, and it doesn't move your team along to place blame and argue. I'm really grateful we did this project because it was very hard and worthwhile. It was a great use of three days!
I learned how to use different data to get answers. Also, we have to see a pattern. This Tastea assignment was really fun. I enjoyed it and look forward to another. Teamwork is really important even though people can't agree, you got to support it. If your group gets it wrong, but your answer was right, you can't blame someone or put them down because probably they will get some right for you. So always stay positive to your teammates and encourage them.
What the Kids Thought
Recently Dan Meyer asks Mathalicious which of these three questions is "real world"?
Karim Ani, founder of Mathalicious, and others have opined without consensus on this particular question and on the general notion of real-world vs. fake-world problems.
I wonder what my 8th grade geometry kids think of this question.
I give them Version A on a strip of paper and ask them to work on it alone for 5 minutes. I tell them that I'm interested in learning if they understand the question as is, therefore I'm not answering any clarifying questions about it. After the 5 minutes, I put them into random groups, and they work on the problem for another 10 minutes.
Then I show them Versions B and C and ask for their preference and reason for each version.
Version A: 18 likes, 14 dislikes
Highlighted reasons for LIKING:
It gives every detail you need to know. It tells you directly all of the information. It also seems easiest to solve.
It isn't as confusing as looking at fast-motion pictures of a circle and a square. Doing math is more exact than visual guessing. [Did he translate the animation to mean guessing?]
I am able to make my own diagram and I can try to solve an equation to find out the answer.
It is simple and not confusing. It allows me to think the way I want to and not be misled by a moving picture.
With the given information, you could construct the two shapes by working backwards.
There's enough given information to make the problem interesting and hard.
I like this one the most because you can actually read the problem and refer back to it.
I think it can be solved using an equation, and be solved more easily than B and C.
It's a more accurate way to find their areas and make them equal.
I like this version because I understand the problem.
Instead of a picture of it on paper, you have to visualize it in your head first.
You can get an exact answer. It is challenging.
Version B: 18 likes, 14 dislikes
Highlighted reasons for LIKING:
It would be cool to build the animation in GSP and solve it that way.
It's a lot more simple. It provides an image and idea of what it looks like.
I can visually see when they are equal. It will be easier to see when they are equal instead of having to do a load of math.
It is visual.
I feel I have a higher chance of answering the question with a right answer.
You can easily see when the shapes have the same area.
Version C: 15 likes, 17 dislikes
Highlighted reasons for LIKING:
It seems easier because you can just count the candies and see if they're equal.
Anyone can count how many candies there are, then subtract the extra space to get the correct area.
Some students like and dislike more than one version. My takeaway on their responses:
Version A
LIKES: (see above)
DISLIKES: Not understanding the question, or "I'm a visual learner, so I like Version B better."
Version B
LIKES: It's visual. It's easy.
DISLIKES: Too fast and hard to follow. One student, "The movement is distracting and confusing. I feel like it's too abrasive and violent. Math should be more elegant than this."
Version C
LIKES: You just count the number of candies. It's visual.
DISLIKES: Too fast to follow. It seems too easy. There's space between the candies. One student, "You can't get the exact answer... And the leftover space in one shape may be more than the leftover space in the other."
I collect all their papers before telling them which version I like. I like Version A for its simplicity. I'm curious if the stated question is enough information for them to understand. This student's reason nails it for me: "It allows me to think the way I want to and not be misled by a moving picture."
We all have students who struggle with word problems. I don't think this means we should give them fewer word problems. I think it means we should give them better word problems — ones that are written with just enough information and not embedded in contrived contexts that either confuse or insult the students. And for students who need help with the question, they get to hear an explanation from a classmate.
Version B is okay, but I don't want to start with it because I feel I'd be wasting a perfectly good question in Version A! I'd reach for a piece of string to explain this question, if needed. Version C gives me a headache.
At least one person in each group understands the question, and they do their best to make sense of it in just the short 10 minutes that we have. They're trying. And making mistakes.
Our whole-class discussion at this 8th grade level:
That "arbitrary" point P is pretty darn close to the middle of A and B. You can roughly tell from using a piece of string. Or you can tell from arriving at y2/(4pi) = x2/16 (where x is distance AP forming the square's perimeter, and y is distance PB forming the circle's circumference) — the denominators are almost the same.
Likewise, P cannot be at the center because pi doesn't reconcile nicely in the equation.
We can solve for x and y using some arbitrary distance AB, and we find y to be slightly shorter.
We can ask a related question: A circle's circumference and a square's perimeter are equal, what is the area enclosed by each? Kids can certainly think about optimization and do a little bit of calculation outside of a formal calculus class.
In addition to asking the students which versions they like, I also pose Dan's exact question to them: Which of these is a "real world" math problem? Or is none of them a real-world math problem?
Their answers vary as widely as those of math educators'. However, I find this correlation that doesn't surprise me: kids who like math more do not care if the problem is real-world or not.
This [Version C] is the most "real-world" solely because of the fact that it involves a material object which in this case is the candy. However, the thing you're solving for in this question is not very "real-world" at all. Personally, I don't care at all if a problem is "real-world" or not; I just like to solve problems.
If a problem didn't have to do with "real-world" I will still do it if I like it. It doesn't really matter.
I don't think any of these problems are "real world" math problems. I like how they make me think. But I don't think I need them in the "real world."
I wouldn't care if it is a real-world problem because I was there to learn. I think all versions can be a real-world problem because it can be needed in some situations.
I feel like all of these problems are real world... But honestly it doesn't matter at all to me. It doesn't matter if it's real world or not, it doesn't affect me wanting to solve the problem.
So, one from the kids.
Teaching Absolute Value
I know Common Core does not have absolute value in grade 8, but I'm teaching it anyway because we're still doing "algebra 1" this year. (A year ago Raymond Johnson looked into the inclusion of this topic in the different grade levels.) My 8th graders know that the absolute value of 5 is 5, and the absolute value of -5 is also 5. Some recall that it's the distance from 0 on the number line. We begin by solving a few of these: abs(x) = 4, abs(a) = 0, abs(w) = -3. A few trip up on the last one but recover quickly and move along.
Me: What is the distance between these two points?
Class: Eight.
M: How did you get eight?
C: Subtract.
M: What about this one? The points are at -3 and 9.
C: The distance is twelve.
M: This one?
C: Thirty-two.
M: Good. Distance is always positive... How did you find the distance between the points again? What operation did you use?
C: Subtraction!
M: Then I'm going to add an equation below each number line showing subtraction. Is that okay?
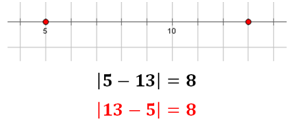
M: So, the distance between 5 and 13 is 8. Then, what is the distance between 13 and 5?
C: Eight
M: Woah! It's the same? Meaning I can write the equation either way?
Kids agree that subtraction can be commutative when it's inside the absolute value bars because we’re just measuring distance. The distance from Johnny to Julie is the same distance as from Julie to Johnny. I'm not going to argue.
Me: Given two points, you can tell me the distance between them. So now I’m going to give you just one of the two points but tell you the distance between them, and you find the missing point x.
C: x is ten.
M: Yea, ten works. Let's try to read this open sentence. How would you say it?
C: x minus six... The absolute value of x minus six is four.
M: Hmmm. Oh, you say the words 'absolute value' because they're there. Let's try again without saying those words. Use the word 'distance' instead.
C: The distance of x minus six equals four.
M: Let me show you again the first one that I'd asked you. I remember just asking you, 'What is the distance between 5 and 13?' What did I not say even though it's there?
C: Minus.
M: Right. Let's not add stuff we don't need. You know naturally that finding distance implies subtraction. So, say the equation again.
C: The distance between x and six is four.
M: Or you could say...? Can we switch the points around?
C: The distance between six and some point x is four.
M: Alright. Is 10 the only answer for x? We are trying to find a point on the line that makes the equation true. So, let's use the number line to solve this. Because we know 6 is one of the points, let's locate it. We need to find the other point that would be a distance of 4 away from 6. So, it could be to the right of 6, or to the left of 6. Where does this put us at?
M: Oh, why isn't the point -6? I see a 'minus six' in the equation.
C: Remember, that minus is for subtracting. We need it there to find distance.
M: I remember. We need it.
We do a few more of these. Enough to bore us, need something new.
M: Let's try this.
C: No subtraction sign.
M: And you said we needed it. Then create it. Make it happen without changing the problem of course.
C: Change it to minus minus...
M: What does this problem say now?
C: The distance between x and negative eight equals five.
We do a few more of these. Enough to bore us, need something new again.
M: What about this?
M: Nothing terribly exciting. The other point(s) that we find is now worth 2x, so we just need to solve for x.
Then we do a batch of these:
Hey, what about these, where there's more stuff stuck around the absolute value quantity. Oh, we just need to first isolate the absolute value, then it's business as usual.
We spend the next whole class using Desmos to explore the shifts/changes to the parent absolute value function. Students need to write down their predictions first before graphing. One student was very excited when she got the V-shape to turn upside down.We discuss some real-life scenarios that may involve absolute values: margins of error, ranges of measurements: distances, scores, speed, temperatures, pH levels, elevations, etc.
Not proud to admit that I spent a lot of hours in college playing pool instead of studying, but never once did I associate the path of the ball as an absolute value function. Consider me odd if I always thought of angles instead.
Solving absolute value inequalities start similarly enough. Kids know from graphing inequalities that there's "shading" involved. They also know the difference between open and closed points. So I just have the kids use their thumb and forefinger to indicate the distance between the two points, then if the inequality says less than [or equal to], then it's natural to pinch their fingers closer together, indicating that the region inside the points need to be shaded.
The textbook will tell kids to set up the "two cases" to solve these inequalities (same thing with equations).
Then, kids are asked to graph the solution. But if kids learned to solve using the number line itself, then there would be nothing to memorize because they learned distance way back when they started learning to crawl. And since the graph shows the solution, then writing what that solution is is easy because it matches the graph. Like below, x lives on the green line between -8 and -2, being ≥ -8 and ≤ -2.
For greater than inequalities, the student would naturally spread his fingers apart to indicate shading outside of the points.
I don't know why there seems to be a lot of rules when learning to solve absolute value equations — which inequality sign for when it's and or when it's or.
Barbie Bungee Revisited and Better Than Yours Class Lists
This year I've taken away a lot of my step-by-step instructions for the Barbie Bungee activity that I'd posted 1.5 years ago. They get no handouts, only some verbal instructions:
[Pointing to the ceiling] See that gob of tape up there? That's leftover tape from previous years where Barbie had taken her jump. It should be at 3 meters up. Well, a small ruler will come out perpendicular (somehow) to that pole where the tape is, and that's Barbie's jumping platform. The ruler is like her diving board.
The goal is to give her the most thrilling jump — her head dips as close to the ground as possible without actually touching it. Yes, her hair hitting the ground is fine. Her jump line is made of rubber bands tied together with slip knots. (Why must we use brand new rubber bands?) You'll work in groups of three, says Instant Classroom.
So, aside from the Barbie doll, what do you think your supplies will be?
Rubber bands! How many? Lots! A hundred!
Try six. Actually seven, but one must be completely wrapped around her ankle, like this.
With only 6 rubber bands, your job is to figure out how many more rubber bands she'll need for the most thrilling jump from 3 meters.
Can we weigh her?
This is like the Vroom car!
So we have to graph, then do the extension thingy. Extrapolate. Oh, the equation is in slope-intercept form!
(We've been looking at word problems and writing linear equations that would be more appropriate in standard form or in slope-intercept form.)
Your team will have until the end of tomorrow's class time to submit your number of additional rubber bands you'd want.
For easier management of the rubber bands, I get them ready in bundles of 7, one to each group for testing and data gathering, and in bundles of 10 and extras to give out as requested on jump day.
I liked the messiness of their initial work. (I didn't give a handout or many instructions for Vroom! either, and they did fine.) Kids doing whatever they think they should do, measuring incorrectly, plotting ill-looking graphs, talking and criticizing one another. I was debating when I should intervene, but it was good for me to just observe and listen in.I waited until the next day to point out stuff. Actually I never told them what they should do, I tried instead to ask them how something should be done. I don't think one single idea came from me — someone always had the answer I was hoping for, so all the "correct" ways to do things came from them. My phone apparently didn't have enough memory after this one clip. It was fun. (One kid also brought up that this was like the Stacking Cups lesson that we did.)
This might seem to you a DUH! share, but I only thought of it earlier this year, and I feel like I invented the paper clip. We all have class lists, of course. But is each of your class lists on a strip of paper like this? And in different colors? I didn't think so.
I have semi-thick stacks of these to use for just about everything. What a pain to write down kids' names for this and that. Instead I just pull out a strip and highlight so-and-so's name and note the reason.
I staple one set of strips together, put a date on it, and kids pass it around to each other to sign in for after-school help — they just need to put their initials next to their names.
Those who need to come in at lunch recess get their names highlighted on the strip.
I use it as a hall pass when I need to send 2 to 3 students at a time to the library.
I highlight a kid's name whose parent I need to contact, then use the back of the strip to make notes from our conversation.
It's a great tally sheet for whatever during class.
Endless uses.
Hotel Snap
[NCTM Illuminations has my blessings and thanks to re-write and feature this lesson on their site.]
Andrew Stadel and I recently presented this task at the 2013 CMC's North and South Conferences.
The Challenge
As a team, build a hotel that yields the highest profit [score].
Rules and guidelines for building the hotel
Each cube represents a hotel room. All 50 cubes must be used.
Hotel must stand freely on at least one side of cube. Here’s a non-example because it’s tilted.
Entire hotel is one piece. A non-example due to yellow cube not attached:
All rooms must have at least one window, a window is any exposed vertical side of cube. The white cube below has no window.
Building costs and tax (daily rate)
Land costs $400 per square unit.
Land refers to outline of top view of building.
All enclosed land is charged, for example, squares marked 8, 9, 10, and 11 in the left outline are open space, but because there's no access to the outside, you are charged for these 4 square units.
A roof costs $10 each, roof is any exposed top side of cube.
A window costs $5 each.
Tax on height of building is calculated by multiplying the tax rate for the highest floor by the total land cost.
Floors 1-10 —> 50%
Floors 11-20 —> 1000%
Floors 21-30 —> 2000%
Floors 31-40 —> 3000%
Floors 41-50 —> 5000%
Income from each type of room (daily rate)
The more windows, the more income.
4 windows, 1 roof = $600
4 windows, 0 roof = $500
3 windows, 1 roof = $300
3 windows, 0 roof = $250
2 windows, 1 roof = $200
2 windows, 0 roof = $175
1 window, 1 roof = $150
1 window, 0 roof = $125
Scoring
Your net profit/loss income will be checked for accuracy. A deduction of 50% of your error will be applied to the actual number. For example, your building nets a profit of $13,500, but your group submits a profit of $15,000, therefore you're off by $1,500. Then 50% of this error ($750) will be deducted from the $13,500 to give your team a score of $12,750.
If your calculations are right on, then your team's score will be awarded an extra $1,000.
Adapting this lesson
Change the number of cubes, as few as 10-15 cubes for younger kids, and maybe up to 100 cubes for high school students.
Also for younger kids, have the Excel file (more on this later) readily available on computers so kids can go back and forth between checking their profit margin and tweaking their hotel rooms — so no calculations needed on their part, they just need to be able to know how to count the different types of rooms.
Older students can create the spreadsheet, it's great practice for understanding how cells work and formulating equations.
Adjust the time for individual and group work based on your expectations.
Modify, take away, or add to the rules and guidelines.
Change any of the costs/income/tax numbers.
Change how you reward accuracy or penalize mistakes.
Ask each group to estimate and rank the profit margins of other teams' hotels just by looking at them (like on a -5 to +5 scale, -5 for biggest loss and +5 for biggest profit).
Ask, "What if all costs and tax stay the same, but now the incomes for the rooms are all reversed so that 4-window-1-roof earns only $125 while 1-window-0-roof earns $600? How would you build your hotel using the same rules?"
If I were to do this with my 6th graders, I'd first have everyone build the same 10-cube hotel with me, then we'd use this hotel to familiarize ourselves with the different types of rooms and tally them up. We could calculate the costs and income together for practice.
How I ran the lesson with 8th graders
(I did this lesson with two classes of 8th graders, one geometry and one algebra. We have 57-minute periods. This lesson took 2.5 periods. I'm so scripted here because the one thing that kids wished they had more of was time. Your teacher instruction needs to be tight and supplies distribution needs to be efficient to allow for all the student work time needed.)
State the challenge of task.
State the rules/guidelines.
Give each student a zip bag with 50 cubes — but I first took time to show them that how they see the cubes now is how they need to be put away when we clean up.
Give each student this cost_income_sheet:
Set timer for 15 minutes for individual work, reminding students that later they will be randomly assigned into groups of 3 to work on one hotel.
While students are working, I use Instant Classroom to put kids into groups of 3.
When the timer goes off, I give the following instructions:
I now need you to listen to directions for working with your teammates, and when I'm done giving these instructions, I will set the timer for 25 minutes for you to work. During this time, you'll need to do the following:
Share and discuss the best model to represent your team's hotel.
You may modify this chosen hotel, or you can even start from scratch, but watch your time.
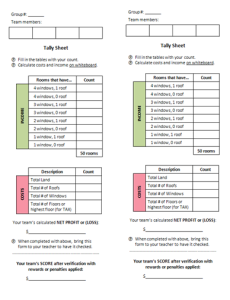
Use this tally_sheet to record your room counts, land, roofs, etc. — remember this is just a tally sheet, so you want to do all your calculations on whiteboard.
On the large whiteboard, divide it into 4 quadrants, and your group will need to fill in 3 of the 4 quadrants answering these questions. Watch your time carefully because when the timer goes off, the hotel you have in front of you is the one you must keep as is.
Allow a couple of minutes for groups to get supplies and settle down together with their individual hotel models. One tally sheet needs to be passed out to each group.
Timer is set for 25 minutes. Monitor the groups, check for understanding, and listen in for building strategies. Keep counting down the time every 5 minutes.
When timer goes off, ask groups to break down the hotels that were not selected and put the cubes neatly back into the zip bags and return to the front.
Next day...
Kids get back into their groups with their whiteboard and hotel. Some groups may still need to finish filling in their tally sheets and whiteboards.
The question for the 4th quadrant is now asked: "If you could relocate just 5 cubes on your hotel, where would you place them?" Remember that you are no longer allowed to change your hotel, this question is just a what-if scenario.
Allow 10 minutes for groups to finish filling in their tally sheets and answer all 4 questions on whiteboard.
As indicated on bottom of tally sheet, groups are asked to bring up the tally sheets to teacher to check their calculations and arrive at the final score.
The Excel sheet
I have two files: one locked and one unlocked. The "locked" one so of course no one can inadvertently change the cells, especially wise if you give kids access to the file to use on their own - only the blue cells to enter data are open. The "unlocked" file is so you can edit as you please.
Notice that all the cells appear in the same order as the tally sheet, makes for quick entries with instant results. My husband gets most of the credit for this, I still claim credit for making it simpler.
Math Practices
I found this form 8_MP_questions_to_ask online and can't find it again to cite the source.
I'm making good use of this form after almost every task now. Students do this for homework. I ask them to highlight the questions that came up during the activity - from teacher, peers, and self.
We discuss this as a whole class the next day and generally come to a consensus of which math practices our task had fostered.
Reflections
We presented this lesson because it went really well in the classroom. The kids were completely engaged. They collaborated, talked a lot among themselves about what they were building and thinking, but they were pretty much dead silent during the initial individual work time. I love seeing the structural varieties — this was true too among the hotels built by math teachers.
To not spoil the fun, I won't post pictures of the high-profit hotels here, but I'd love to learn what some of your profit numbers are from your class.
Vroom Vroom
I normally share a lesson that I've already done with students, but I'm still tweaking this one and needing to write some thoughts down before my noggin turns back into soft tofu. I bought these 14 pull-back friction toy cars (2 sets) for $30 at Costco. Amazon posts a video of how well these soft squeezable cars can stand up to toddler abuse.
On your mark...
Each team of 3 students gets 1 car.
How far the car moves forward depends on how far it gets pulled back — good lesson too for talking about potential and kinetic energy.
The challenge is to get your team's car to go a certain distance (reach finish line) without going over. This distance is not revealed until later.
To predict for how much pull-back a car needs to go a certain distance, students "test drive" their cars for pull-backs of 2, 4, 6, and 8 inches.
Get set...
Students are instructed to take measurements from the car's front wheels.
The blue tape marks the starting line. Front wheels line up at the front of the tape.
The car is then pulled back a certain distance — here it's 2 inches — and let go.
When the car stops, the distance traveled is measured from front of wheels to front of blue tape. Looks about 13.2 inches here.
Go!
Teams do 3 trial runs.
Record and graph data on Desmos.
Now teacher reveals and marks the distance each car needs to travel without going over. Say 15 inches.
From graph, students extrapolate what the pull-back distance would need to be for car to move forward 15 inches. This distance is recorded and cannot be changed.
Teams line up with their cars — one at a time — to pull back and let go!
Winning car is one that reaches closest to finish line without front wheels going past it.
Considerations
The cars roll best on bare floors. My classroom is carpeted, so we'll need to do this outside.
It's fun that the cars actually vary in speed for same pull-back distance. The sports car (bottom left in above picture) is fastest.
My kitchen floor is not big enough to test longer than 8-inch pull-backs, but the graph appears more parabolic.
The data looks pretty awful, doesn't it? Kinda? Maybe?
Ginny from Mathalicious was in the kitchen when I was playing with these toy cars. She shook her head and wondered if empty nest syndrome got the best of me. No matter. I think this will be a lot of fun. Will report back and post some pics.
[Updated 12/01/13] Jon Orr did this lesson with his Grade 9 students and wrote a great summary on his blog.
Here are a few pics from my class:
And a couple of video clips:
Deconstructing a Lesson Activity – Part 2
Previously: Deconstructing a Lesson Activity - Part 1
No matter what I write in this Part 2, I hope it's not a wrap-up of this topic. On the contrary, I hope it opens up and extends our conversations on improving the implementation of rich tasks in our classrooms.
Physical arrangements, whiteboards, groupings. The student desks are set up in rows and columns in my classroom. Not terribly exciting, but don't judge a teacher by his/her furniture arrangement. When I'm given 38 desks (I had 37 students in Math 6 last year) and x square units of floor space, my creativity is stifled.
Work with whatever space you have. Push the desks together, pull them apart. Kids don't mind sitting on the floor. (If they do, ask them to stand and see if they like that better.) Can they work in the hallway — or outside if you're in warmer climate — where you can see them from inside the room? Just make sure you are constantly roaming among the groups.
Big-ass whiteboards. Nathan just wrote a letter asking for these. Last summer I sent my principal the link to Frank Noschese's post — and the whiteboards were waiting for me when school started. I got ten 2' × 2.6' boards, each at $10.50 from here. Please get them. By far the single best school purchase, worth their weight in gold. Just how much do I love these? You touch my whiteboards, I'll kill you.
Randomly assign kids to groups. We do group activities often enough that eventually pretty much every kid ends up with somebody new in the group. If you try to group them "heterogeneously" with high-medium-low kids, then you accomplished just that — you just told them who's high, who's medium, and who's low without saying a single word. Kids aren't stupid. I use Instant Classroom to randomly assign. You can always use your discretion to change a few kids around after the computer picks them — but still let the kids think that the computer did all the choosing. I never heard any whining. Kids don't whine at what the computer says.
Group roles. What are these?? (No, I'm asking you!) Like "facilitator," "recorder," "reporter," "budget person," "dietitian," "hairdresser," etc... These roles wouldn't work with problem-solving tasks. I don't want a kid sitting there doing nothing because it's not time for his role to occur yet. Please, no assigned roles. Except the one about trying to solve the problem.
Grading this type of task. I don't see dead people, but I hear student voices all the time. While I enjoy grading as much as I enjoy poking needles in my eyes, I hold certain beliefs about grading problem-solving group tasks (and the student voices that guide mess with me). And my possible reasons/solutions for them?
It is wrong to give a lower grade because they socialized too much instead of focusing on the problem. (We're teenagers and you expect us not to socialize? OMG! Did she just put me and Joey in the same group? He's sooooo cute. How's my hair? This problem is just too hard anyway! We really tried but we got stuck and you were too busy with another group to help us. Laura is such a show-off. I wish Andrew would grow up.) If the whole group is off task, then I'd seriously reconsider the relevance/engagement level of the task and the social dynamics of the group. It's back to that Step 0 of picking the right task that's engaging and has low entries so everyone can get on board. It's my fault that the kids are not on task.
It is wrong to give a lower grade because they did not come up with the correct solution when the bell rang. (Sucks that we didn't win the game today, but we still had a good game, right? Didn't we work well together as a team, especially on defense? Nice block there, Mitch. I almost had a pick right there if my damn leg didn't cram up! Ha, now I know what Coach meant by the hook-and-lateral play!) Our goal of wanting kids to engage in problem solving is to honor the process that they go through — their thinking, their collaborating, their critiquing one another. We want to tap kids' two most abundant natural resources: their curiosity and their need to socialize. I simply cannot justify putting a grade on this.
But bottom line, you grade it if you want to. Don't grade it if you don't want to. I graded fewer than 50% of the tasks that were done in class last year. When I did "grade" them, I gave full credit. To worry about how to grade group tasks is really to sweat the small stuff. That said, if you had a handout for each student that went with the task, then it's fair to give the individual grades.
Establishing a classroom culture of problem solving and finding time to do so. Stephanie Reilly's question in Part 1 helps me shape what I'm trying to convey in this section.
I can't think of a better day to start doing problem solving with kids than Day 1 of school. Kids pick up on what we say we value and what we do to back that up. Set a goal to do one task every two weeks. Too ambitious? Then once a month. Just please don't give up. On Day 1, I might just start with Pyramid of Pennies (Ha! I nailed the spelling there) or the new Bracelet Craze problem. If I were a student and knew that all my teachers would go over "Rules & Procedures" on the first day of school, then I'd be tempted to feign high fever and induce vomit to stay home.
However, you need to come up with guidelines for group work that you will share with kids before they begin. Culture takes time. It takes a lot of reminders too. I'll share what I say [for guidelines] to the kids under "group time" in the last section of this post about implementation.
Post the strategies for problem solving in your classroom. I have these on just regular size paper, but laminated, and we refer to them all the time. You know, strategies like these ones.
Teacher concern: I'm afraid I don't have time to do this because there's still so much to cover in the textbook. You can't do this and feel guilty. (Remember how crazy in love you are supposed to be with the tasks you choose for them?) You have to be okay with not being able to go cover the textbook front to back. The person who tells you that you have to do so is delusional and mean. Common Core does have fewer domains and standards at each grade level. Spend this summer mapping out key concepts and lessons. I believe in having some sort of pacing guide, but I don't believe in having it dictate how we move through the year — the kids and your formative assessments of their learning should govern the flow. I haven't done research or have hard data of my own to give you, but I believe your kids will do better on year-end assessments if they have been exposed to problem solving throughout the year. Trust me? :)
Carve out time by re-examining and possibly eliminating things that you normally do.(For the last two years our students had two periods of math each day. I think this is going away next year, so I have to re-think this through too.) Besides just having better classroom management — meaning it's not taking you 10 minutes to get the kids to settle down and start class — how effective is your use of class time when you do these items?
Warm-ups
Games
Review games before a test
Pre- and post-surveys
Benchmark tests (beginning, mid-year, end-of-year)
Stuff that kids can do at home blindfolded (I think we know what these are.)
Class parties (What the hell are these? I like parties too, but let's have them at lunch time.)
Lastly, please don't forget that these are perfectly good SCHOOL days for doing mathematics: First day of school, last day of school, last day of the quarter, first day of the quarter, whatever day. The day before Christmas or spring break. The first day back from an extended vacation. Sub days. Your sub is perfectly capable of passing out a meaningful handout (it's meaningful because you made/selected it), and it will go well because you have already pre-taught the kids what's on that handout — give them a sneak peek at it! — and shared with them your expectations the day before you leave. If there's one assignment worth grading, then it's the work that they do while your sub is there.
Finally, implementing the task. Thus far I've covered the behind-the-scenes stuff that was missing from my lesson posts. The task itself is actually a lot more straightforward — pretty much what you read on my lesson posts is my best storytelling of what went on in the classroom.If you're doing an actual 3-Act lesson a la Dan Meyer, then you're good to go! These are some of my favorite 3-Acts that I'd written up:
File Cabinet
Taco Cart
Equilateral Triangles
Penny Pyramid
So, this is an outline of how I implement a non 3-Act problem-solving task.
Ask for a volunteer to read the problem aloud (5 minutes). Each kid gets a copy of the problem to follow along. After it's read aloud, everyone reads the problem again quietly to self. Then my questions begin for the whole class:
What are we trying to solve for in this problem?
What information do we know?
Is there information that you wished you knew? Why is it not given then?
What's the first strategy you have in mind that might help you attack this problem? And why did you say 'do a simpler problem'?
Depending on the task, I've also begun to ask — instead of the questions above — these two questions from Annie Fetter (YES! Please watch the 5-minute video if you haven't.) The kids write down their answers, and I randomly call on them to share.
What do you notice?
What do you wonder?
Quiet individual work time (10 minutes). You have to allow for some individual thinking time. I can't work on a problem when others around me are talking. So I set a timer for 10 minutes. Of course it doesn't have to be 10 minutes, it's up to you and depends on the problem, but this is NOT the amount of time in which I expect any kid to solve the problem. If a kid does solve it quickly, then hopefully you have an extension — you should always have an extension — ready for this kid. If a bunch of kids could solve it quickly, then you've chosen the wrong task, too easy. Back to Step 0.
I say something like, "I'm setting the timer for 10 minutes so you can think about and start the problem on your own. There's no talking and no sharing at this time. You'll get in groups to continue to work on the problem after the quiet time. You're welcome to get up without my permission to get any tools (protractor, compass, ruler, graph paper) that you need. Do you have any questions for me before you begin? Remember our rule of NEVER TELL AN ANSWER. Go!"
While they're working, I use Instant Classroom to form the groups and move some kids around if necessary.
Group time (30 minutes). Again, this is a very generic time allowance. You're the teacher, you'll know by how much to shorten or lengthen the time depending on the groups' progress or lack thereof.
I say something like, "You will now continue to work on this with your group mates. You will use the large whiteboards to show your work. Everyone has his or her own marker to use. But now I'll explain more by what I mean by 'never tell an answer.' If you think you have an answer already from working on it just now by yourself, then please don't share it with your group. Choose to be the last person in your group to speak because I actually need to speak with you first.[1]
... Also, every time we do a task and I hope we get to do lots of them, I ask the computer to randomly assign you in groups, so if you have a complaint, take it up with the computer. If your group would like more individual work time, like 5 more minutes, then that's great and fine by me....
I'm interested in your working together to solve this task. I'm not asking you to become best friends. One person speaks, everyone else listens. Argue about it, but be respectful. Ask questions of one another. Don't take so-and-so's word for it, ask him or her to explain it. Don't let others think for you. Help each other out. Maybe this whole structure is new to you, don't worry about it. I'll walk around and listen in and smack you in the back of the head when you don't quite have it right. Just kidding. Not. Yeah, I'm kidding. Go!"
I actually repeat much of this same spiel throughout the year.
[1] So I talk privately to the student who does have the correct solution and suggest a few things:
Can you solve the problem a different way? (It's important that you do not force a student to find another strategy especially when you can see that she has found the most elegant one already — this just seems counterproductive to me.)
I'd like you to try the extension to this problem. What if...?
I need you to go back to your group and practice really good listening skills. I just want you to listen to your teammates talk. Then see if you can help them by asking questions only. Kinda like what I normally do with the whole class. You may give them one hint if they're really stuck. You want to give that a try?
Teacher role during this group time. This is where the book 5 Practices comes in for me. I've been presenting its contents at workshops over the last two years. There's no way I can do it justice here, and I've already written a brief post on it just as a quick review.
The gist of it is that I go around and listen in and check on the groups' progress. I ask questions of specific individuals in the group.
Hey, Julia, can you explain to me what I'm seeing here on the whiteboard? Maybe you didn't write it, but whoever wrote it, did he/she explain it to you?
Jonathan, I'm not sure where this equation/number comes from. Please explain.
I saw this same strategy at Erika's group. Allie, did you come up with this strategy? If not, what is yours? Where is your understanding of the problem so far?
Joey, what has Cindy contributed to the group thus far? (If Joey says, "Nothing," then I'll ask Joey again, "What have you contributed?" I don't remember ever having two people in one group who have not shared anything. Remember they had 10 minutes of quiet time to work on this already. They have something to share!)
Cole, your group is over here. I don't want to tell you that again. (And I never have to.)
Now, when there's one group that has made a lot more progress than the other groups, I ask for the whole class attention and say, "Julia's group has made an important connection, so I'm going to ask someone from her group to share with the class one hint, one strategy, or one something that would help all the groups along. Listen carefully."
If none of the groups has made progress, then the teacher needs to jump in with a hint. But be patient too!! You have to watch the clock. How much time is reasonable? Are the kids mostly working and asking questions of one another? If they're exhibiting productive struggle, then let them be. Nobody is going to die if you extend this lesson another day.
The SHORT version can end here after the groups have figured out the solution. Maybe not all the groups finished, but remember, most of them did. Depending on the task, depending on the students, depending on time, depending on whatever you deem as important, you can end the lesson here and not feel guilty that there was no large-group sharing at the end, no connections made among the different strategies. Instead, focus on all the mathematics that you did allow the kids to be engaged in. I see enough teachers feel discouraged that they "didn't get to do everything that I wanted to do" — it's not about doing everything, it's about doing something to get started, to get better, to suck less each day, to remember why you went into teaching in the first place.
The FULL version includes the "connecting" piece that the 5 Practices refers to. It's about making connections between the different strategies, and you accomplish this by having the groups share their work on the whiteboards. (This step is moot if the task didn't have more than one strategy.) Kelly O'Shea is my whiteboarding goddess. And connecting is also about you the teacher making the connections of all their work back to the original intended learning goal of the task.
Who says you can't add the connecting piece to your short version 2 or 3 days from now (hell, even two weeks later) and make it a complete kick-ass full version? In real life we return to problems all the time. Snap a photo of each whiteboard if the kids need to refer back to their work at a later date but you have to use the whiteboards for another class in the next period. Problem solved.
You can do this. We can do this together.
Deconstructing a Lesson Activity - Part 1
[I've decided to break this post into two parts because I don't want to bore and/or discourage you, and I need to take a breather. These two posts in particular are truly my labor of love because if there's one thing that I find myself proficient at implementing in the classroom it is problem solving. I hope you'll find some parts useful.
I've done a fair share of posting actual lessons and pictures from my classroom in this space and on my 180 blog [that's no longer available, sorry]. But I'm afraid they appear polished and therefore unhelpful to teachers who are trying to implement problem solving, white-boarding, 3-Act lessons, or any task-oriented activity in their classrooms.
So here's my earnest attempt to deconstruct the structure of a lesson, get down to the nitty-gritty, take small bites (and spit out what you don't like), and make it real because if it ain't real to you, then it ain't gonna happen for your kids.
Some important prerequisites. I need you to have this mindset or else we're not going to accomplish anything.
You care that kids learn something meaningful in the 45-minute period that they are with you. You might be thinking, Of course I care or else I wouldn't be teaching. No, I don't mean that. I mean the "something meaningful" part. What did you intend for your kids to learn today?
You might very well fail at implementing a 3-Act in your first attempt. And fail again and again. But you can't give up. You can't give up because the kids need you to persevere, it's the same MP1 that you ask of them. Cry and bitch about it at home. Adopt a puppy if you live alone. Eat ice cream. Drink a beverage with higher alcohol content if you need to. Get a punching bag or go to the gym.
You need to be okay with leaving some children behind on some days. I'm not a miracle worker. Neither are you. One hundred percent student engagement 100% of the time is a myth sold by the snakes-oil salesman. You can't differentiate every lesson. You can't reach and motivate every child. But you will reach and motivate the ones that you can. You'll die trying because you love these kids, but you're going to realistic about it.
Surround yourself with helpful colleagues. They listen and are willing to observe your class and give critical feedback. They remind you to eat. They eat lunch with you. They don't badmouth kids when their lips are moving. They believe Happy Hour is invented for schoolteachers and feel it's sacrilegious if they went without you. The toxic people in your life can just piss off. If no one is around and you really need to vent, please email me at fawnpnguyen at gmail dot com. I am a much better listener than I am a writer.
Piece de resistance. I carry out the lessons through the lens and language of these bodies of work. It's okay if you don't have the 4 books, but I highly recommend them if your school or personal budget allows.
5 Practices For Orchestrating Productive Mathematics Discussions by Margaret S. Smith and Mary Kay Stein
Thinking Mathematically by John Mason with Leone Burton and Kaye Stacey
Mindset: The New Psychology of Success by Carol S. Dweck, Ph.D.
12-minute video of Dan Meyer's TED Talk: Math class needs a makeover
Improving learning in mathematics: challenges and strategies (PDF file) by Malcolm Swan
The Art of Problem Posing by Stephen I. Brown and Marion I. Walter (I just borrowed this book from UCSB last week. It's wonderful. Nat Banting reviewed it here.)
Picking the right task. Think of this as Step 0 of the 5 Practices. If I picked the wrong task, then no sound pedagogy or fancy technology would be able to save me. I'm done. Lesson sucks. Game over. That's how important this step is. What is a "right" task?
It's age appropriate. I don't mean for you to go searching under tabs that read "6th grade" or "algebra 1" either. What's age appropriate for your 6th graders might be too high for my 6th graders. Know your kids. An inherently good task would cover a wider range of consumers. If it's early in the school year and you don't know your kids well enough yet, then choose a higher level. It's a crime to underestimate children's mathematical abilities. Dan Meyer speaks volumes about "low-entry high-exit" tasks and Ladder of Abstraction.
It has multiple strategies. At least 2 ways of solving. Single-strategy tasks are like culs-de-sac. There's nothing to do except to turn around. Think how fruitless and boring it would be if you asked kids to share their different strategies and there wasn't one to begin with. Also, just because you'd struggled with a task does not necessarily mean all your kids will. Maybe there was a more elegant solution that you did not see. Be humble, ask another colleague or throw it out on Twitter for others to give it a try. (Please tell me you have a Twitter account. Mine is @fawnpnguyen.) And if your gut thinks there's another way to solve a problem, then be honest with the kids and say exactly that. They'd be thrilled to death to learn that they'd helped you see something differently. My favorite moments for sure.
You are crazy about the task. I can't speak for what tasks/problems turn you on, but I know what my favorites are. (And every.single.time we do a new task, I half-jokingly say to my students, "This one is my absolute favorite!" The kids roll their eyes at me, but they know I'm passionate about it.) It's hard to get kids to like things that we ourselves do not care much for. That's kinda fraudulent. I'm be a big fat liar if I say that I like all the tasks Andrew and Nathan have on their sites. Your chosen task is your baby — you've personally nursed and nurtured it. Kids sense this and they'll handle it with care too.
Throw a curve ball. Meaning offer a task that does not line up with your current topic right now. I know this sounds strange. (I've never read or heard anyone else suggest what I'm suggesting). Normally teachers look for a task that lines up with what the kids are learning. Sure, I do these "tasks" too, but I really call them "exercises." Exercises help you practice the skills you're learning. The tasks that this post is referring to are problem-solving tasks, and true problem-solving has no prior diagnosis and certainly no given prescription. Don't do Taco Cart right when you're teaching Pythagorean Theorem. Unless you want to do it as an exercise, then sure, go for it! The beauty of this is you can reach into your folder of best tasks, close your eyes, and pick one! Be a rebel, break the rules. (Don't forget it still has to be an appropriate level task.)
Custom tailor the lesson. This is hard work. I don't care if His Holiness the Dalai Lama himself wrote the lesson, I still need to tweak it so it works for me and my kids.
Great lesson but terrible handout. I see this all the time. Take the time to re-type it. Is there wasted space or not enough? How's the font size, the heading, the outline? Can you improve on the graphics? Are students asked to work on page 2 but keep having to flip to page 1 to see the sketch or data?
Do you have to pass out the handout at all? How about starting from scratch as in having the kids take out their own paper to create data and meaning for themselves? Remember that any question that appears on a handout may potentially rob a kid's opportunity to ask that question for herself. There are certainly good handouts that have just the right amount of information and provide easier access points for kids. Please create them.
What level of technology is involved? Disaster abounds when an activity requires at least 15 computers and you only have 5. Adapt it or scrap it. No more than 2 kids should have to share a computer. Will the server crash if everyone got on the system? Did you review the YouTube video for all the potential peripheral garbage and comments that might be on there? To be safe, how about you projecting the video from your teacher computer instead? And I need to add here that I beg you not to incorporate technology into a lesson for technology's sake. Technology should enhance the student's learning. A shitty lesson on the interactive whiteboard is still a shitty lesson.