My Other Math Sites
Lessons From the Classroom
Math Worksheets
I often create worksheets for my students, even though every district-adopted math curriculum we've had has worksheets for students. I do this for two reasons:
I sometimes want to teach differently than what the curriculum writing team was thinking.
There's a particular structure/scaffold that reflects how I see the content can unfold for learners.
Here's a sequence of practice questions for my 8th graders on rigid transformations.
Everything about this is intentional.
Item #1 is a completed sample of what's to come. This is a practice worksheet, not a problem-solving task, so I will be clear about what is expected.
I remove certain parts in item #2, while keeping it similar to item #1.
Item #3 comes before item #4 because I think it's easier to follow the stated transformations than to say what they are.
Item #6 asks for more flexibility but with an ending constraint.
Item #7 opens up the problem and allows for peer exchange.
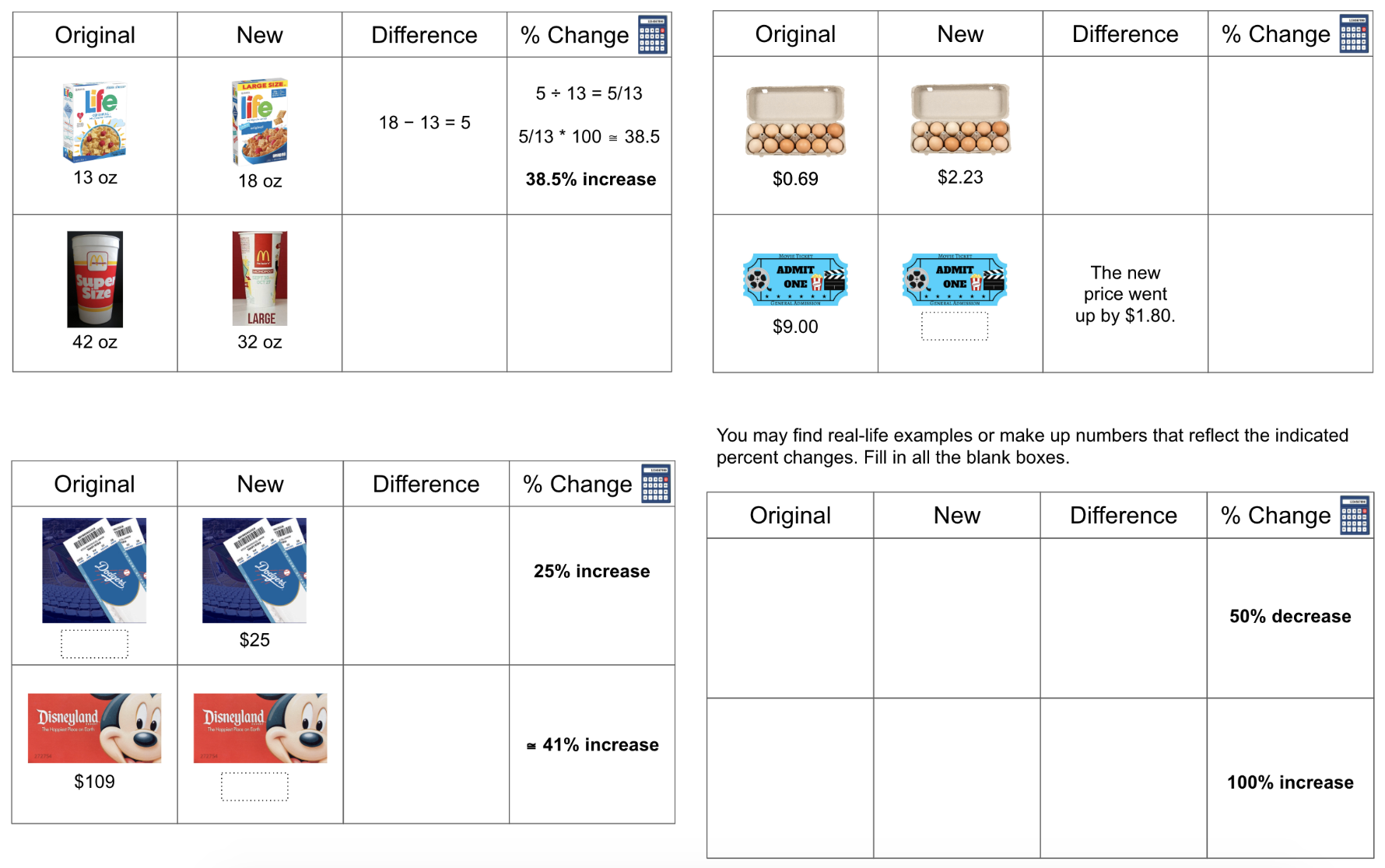
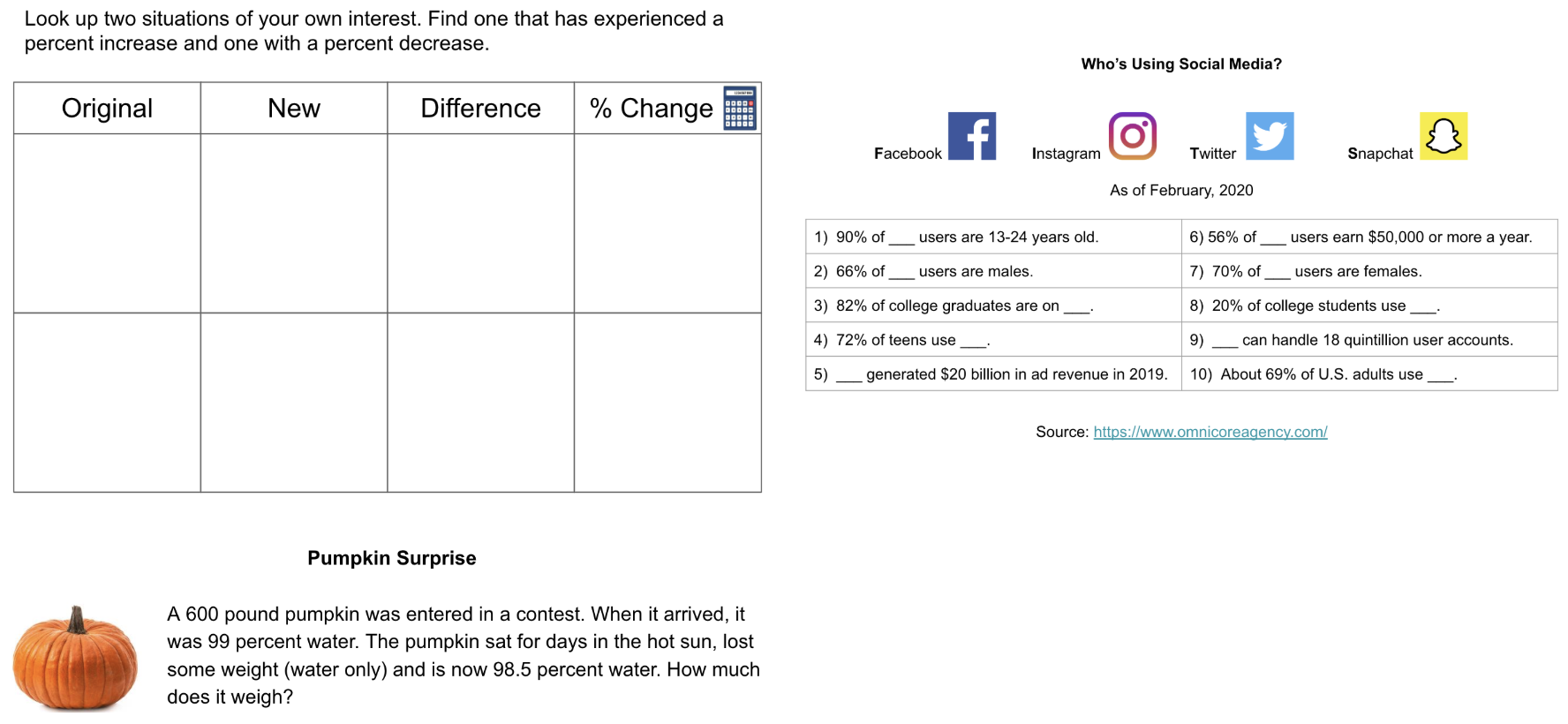
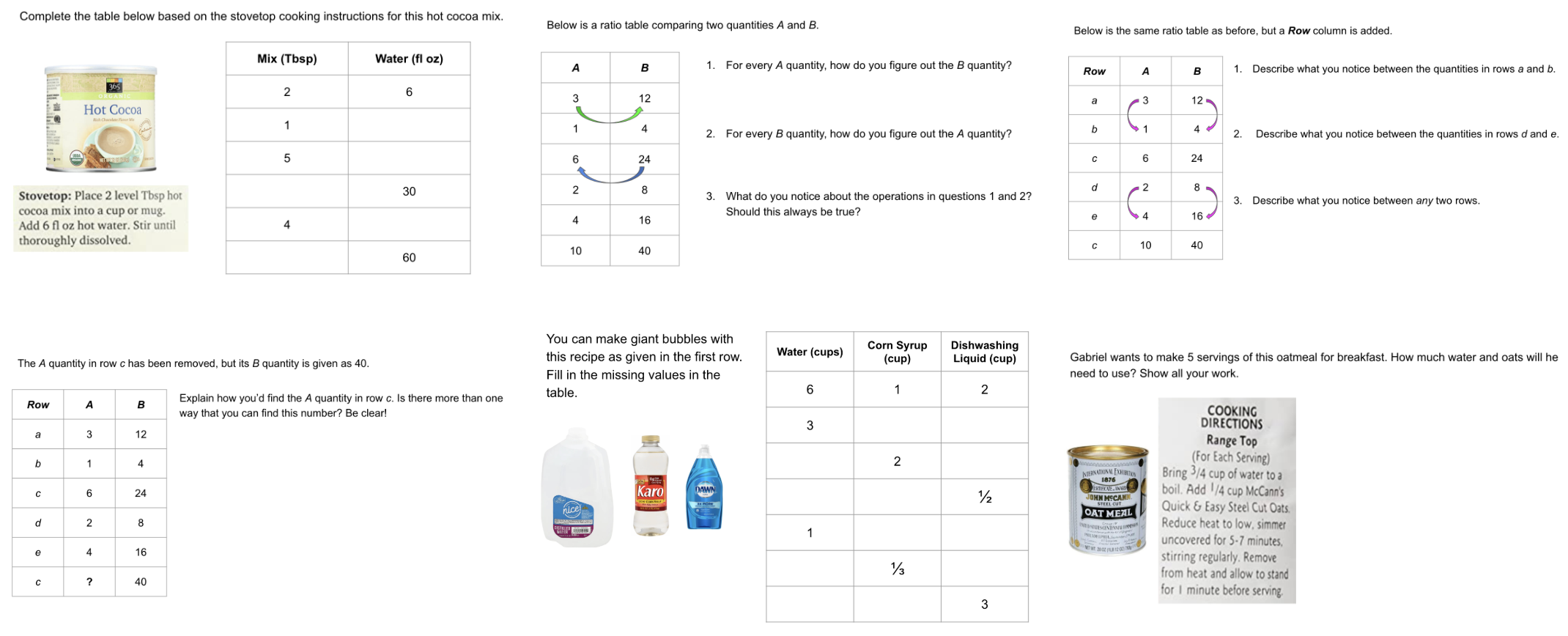
It's esthetically easier for me to create the questions on Google Slides. I then do screenshots to toss them onto a Google Doc. Here's a screenshot of questions for 7th graders on percent change. Here's a screenshot of questions for 6th graders on ratios and rates. If you'd like copies of these:
Yes, each of these takes one unit of shit-ton of time, especially when I have to look up real products with real numbers. But it's an OCD thing too, as in If-I-can-make-it-better-I-will. Stay safe, everyone.
Solving an Equation With a Fraction
From CPM:
The Sutton family took a trip to see the mountains in Rocky Mountain National Park. Linda and her brother, Lee, kept asking, “Are we there yet?” At one point, their mother answered, “No, but what I can tell you is that we have driven 100 miles and we are about 2/5 of the way there.”Linda turned to Lee and asked, “How long is this trip, anyway?” They each started thinking about whether they could determine the length of the trip from the information they were given.
And I like both methods, especially Linda's.
Without using a visual, we may have students solve for x in the equation (2/5)(x) = 100 by multiplying both sides by 5/2.
But I notice two things: 1) Students don't always remember why they are multiplying by the reciprocal, and 2) Students have difficulty showing Linda's method with an equation like (9/2)(x) = 27.
So, I'm having the students think through the problem by answering these two questions:
If we know that nine halves of x is 27, then what is one half of x?
Now that we know what one half of x is, what is a whole x?
As we write the fractions, we can keep our focus on the whole number numerator and treat the denominator as if it were a thing, and that thing is not changing.
Another example,
This helps us go back to finding the unit rate in the first step via division, and then find a multiple of that unit rate via multiplication.
Once students make sense of these two steps and become fluent in solving for a whole x, then they can work on the not-so-friendly equations — such as (5/6)(x) = 4 — because they are more confident and trust the process.
Sure, multiplying by the reciprocal would have solved for x in one step, but there's something uniquely comforting to students when they can first find just one part of something.