"Working Together" Problem

Heya, back-to-back post about a problem from Five Triangles mathematics.

When I tweeted how much I love this problem, a few people did not feel the same at all. Here are my reasons for appreciating this problem:

1. It's a notched up "working together" problem that I have not seen before.

2. It has percentages and fractions.

3. I can use rectangles to solve this. (I was asked on Twitter how I would solve this using rectangles, hence this post.)

4. I had to work on this problem. This is a big reason for me. We should assume that if we're teaching a particular math subject — Geometry, Statistics, or Calculus — that we're able to easily do all the exercises in the textbook. A set of exercises allows us to practice a particular skill. But a problem should require us to think. I hope I've encouraged problem-solving enough with my students that they value a problem more when they have to struggle with it, when they don't know immediately how to start it, when they get stuck and become frustrated, when they seek others for help, when they can leave the problem and come back to it another day.

While I'm at it, I also love the site Five Triangles in general for a couple of reasons:

1. The Geometry problems are simply stated and interesting. They make me pause and think, very few have been automatic gimmes.

2. The solutions are not posted. I really appreciate this because if they were, we might be tempted (mainly due to lack of time) to check the answers too early before we allow ourselves a chance to work through the problem and perhaps struggle with it. "Anticipating" is the first of 5 Practices that gives us insight on how students might solve the problem.

I did, however, retype the question above so it's easier to read and track information. I also numbered the paragraphs for quicker reference.

How we worked through this problem. Colors and all.

Draw a rectangle to represent the task. It has an area of 80 square units because that's the LCD of the three fractions in the problem.

Because this grid represents the task, we use it to fill in the amount of work done. Paragraph [3] is the first concrete piece of information that allows us to do this.

We continue to fill in the work done as described in paragraph [4].

Paragraph [5] is the first piece of information that allows us to figure out C's rate. Knowing that C can do 16 boxes in 8 hours means C can do the task — 80 boxes — in 40 hours.

With C's rate, we can now take on paragraph [2]. We know from the last step that C's hourly rate working alone is 2 boxes per hour or 10 boxes in 5 hours. But when working with A, C's rate is 40% faster, therefore instead of getting just 10 boxes done, C can get 14 boxes done in 5 hours when working with A.

From picture above in green, we know A and C did 24 boxes in 5 hours, and since C was responsible for 14 of those, the remaining 10 boxes were done by A.

Then A's hourly rate when working with C is 2 boxes per hour. Because this hourly rate represents a 20% increase than if A were to work alone, the math we need to do is 2 boxes divided by 1.2 to get 5/3 boxes. Solving for x in the proportion below gives us the answer that A completes the task in 48 hours.

Lastly we use paragraph [1] to figure out rate for B. We know A's alone rate is 5/3 boxes per hour, but when working with B, A's rate is 40% faster. Thus we multiply 5/3 by 1.4 to get 7/3. If A can do 7/3 in 1 hour, then A can do 35/3 in 5 hours when working with B.

The yellow boxes show that A and B can do 25 boxes in 5 hours, so subtracting 35/3 from 25, we see that B did 40/3.

To get B's alone rate, we divide 40/3 by 1.2 (because B is 20% faster when working with A) to get 100/9. Solving the proportion below gives us the answer of B completing the task in 36 hours.

Fraction Division via Rectangles

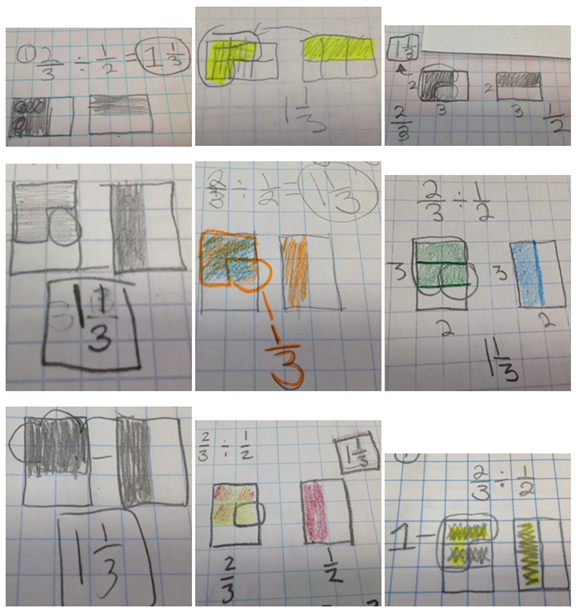
I appreciate Christopher Danielson's post on common numerator fraction division because it's important to examine how various algorithms work and how we can help our students become more flexible with their thinking. It's not surprising that I teach fraction division using rectangles, and I really believe the kids seem to grasp it better because it's visual.

I'll start with this problem: 3/4 ÷ 2/3. But before we do fraction division, I ask kids about whole number division. What is 8 ÷ 2? What is 15 ÷ 5? Eventually we settle on something like: asking what is 8 divided by 2 is the same as asking how many groups of 2 are in 8. Then we apply the same question to 3/4 ÷ 2/3 as "how many groups of 2/3 are in 3/4?" I guide them through this process:

Me: Let's draw out 3/4 and 2/3 on paper.

Half of them draw circles. Awful, drunk, ill-behaved circles.

M: Let's use grid paper instead to draw our rectangles. I think you can show 3/4 much more accurately on grid paper than on a circle. Please draw 2 rectangles of the same size.

(By doing this, we are really dividing two fractions using the common denominator strategy. Christopher writes about it here.)

Students: Any size?

M: What size do you think? Does it matter? Shade the first one to show 3/4 and the second one to show 2/3.

They mess up. They might draw a 1 x 4 rectangle, shade in 3 to show 3/4. But they don't quite know how to shade in 2/3 of a 1 x 4.

M: So maybe we should think about the size of the rectangle more carefully. Look at the problem again. Three-fourths divided by two-thirds. Hmmm... What dimensions should our rectangles have so it's easy to divide into fourths and thirds.

This prompt is enough for someone to say, Draw a 4 by 3 rectangle!

M: Bingo! I'm drawing these with you. Okay, so two rectangles of 4 by 3 — or 3 by 4 — doesn't matter. I'm shading in 3/4 on the first one and 2/3 on the second one. So our question is: How many groups of 2/3 are in 3/4? Because I colored mine in, can you help me ask the question again using colors instead?

Someone responds, How many pinks are in the greens?

M: Yeah. And how many little squares are pink? Okay, eight. So, I'm going over to the green here and round up 8 pink squares. I'm able to round up one group of 2/3 (pink) in the 3/4 (green).

Someone says, There's one left over.

M: How much is this one little green square left over worth? Right! 1/8 because we called 8 little boxes as one, so 1 little box must be 1/8. Our answer then is 1 and 1/8.

A few students say, I get it.

M: How do we know that our answer of 1 1/8 is correct? Okay, we'll use a calculator.

I purposely use an online calculator where I'm entering the fractions as they appear. I don't need to distract them right now with decimals or talk about parentheses. This is from CalculatorSoup.

M: Let's do this again. Now with a mixed number just for fun. Let's do 1 1/2 ÷ 2/5. How many rectangles are we drawing? What dimensions should they be? Oh, but we have more than 1 whole here, so...? We should have something like this then.

They say, How many groups of orange are in blue?

M: So let me round up the groups of orange that are in the blue. I got three. And the leftover is? Right, three. Three out of...?

More students say, Three-fourths! Three and three-fourths. I get this!

This online calculator from Calcul allows for entries of mixed numbers.

M: Okay, your turn to do one all by yourself. Please do 2/3 ÷ 1/2. (Same one Christopher used.)

I think these kids' papers show understanding.

While these are not there yet. I don't know. But it seems that drawing pictures and doing more visual stuff start to disappear in middle school. 

Below is our textbook's treatment of "dividing fractions and mixed numbers" — Chapter 5, Section 7 — the full 3 pages before the Exercises. 

Notice the two circles at the start of the section — that's pretty much it. And circles are great if you have denominators of 2, 4, and 8. I think if I can get my kids to first see the answer, then I can sell them the other algorithms — like multiply the reciprocal — and not come across as a fraud.

I also want to point out that I normally see this visual below for division of fractions. My way is different than this — I deliberately ask kids to draw 2 rectangles whose dimensions are the denominators.

[Update 01/07/2017]

Thank you to Rachel Emily Tabak for creating this accompanying worksheet, 18 - Frac division rectangles

More Rectangles and One Good Read

I just started reading this book on Ramanujan, and I highly recommend it.

Curmudgeon just posted this Painter's Puzzle yesterday on Christmas Day — what a nice gift for us!

A painting contractor knows that 12 painters could paint all of the school classrooms in 18 days.They begin painting. After 6 days of work, though, 4 people were added to the team. When will the job be finished?

Students typically read this as a proportion problem: 12 painters can do it in 18 days, so 1 painter can do it in 1.5 days. Except... hmmm, no.

Edward Zaccaro uses what he calls the "Think One" strategy in his book to solve this type of problem. I guess mine is the same idea, except I draw rectangles. Shocking. :)

Kids are terrified of fractions already. Teaching them to solve this problem — or any of the work problems — using rational equations will only confirm how much they dread the blessed fractions. Sure, I'll get to the equations, but I just wouldn't start with them.

Another common problem — that I'll use rectangles to help my kids — goes something like this:

In a state with 10% sales tax, someone buys an article marked "50% discount.” When the price is worked out, does it matter if the tax is added first, and then the discount taken off, or if the discount is taken off, and then the tax added?

Tax, then discount:

Discount, then tax:

Heya, I'd love to send the Ramanujan book (from Amazon) to the first person to email me at fawnpnguyen at gmail dot com.

[1:49, Robin S. from PA will be receiving the book!]

[3:42, Elaine W. from VT is also getting the book.]

Hope you're enjoying your break.

Area of a Circle

After finding the formula for the circumference of a circle, my 6th graders were ready to work on finding the area of a circle.

I asked them to draw a circle on notebook paper, any size, but not too small. Then I gave each a centimeter cube to trace one face onto their paper to remind them how much the area of one square centimeter covered. (You can see it drawn on top right pic below.)

Question 1: Give me a guess — only by looking — what is the area of your circle, in square centimeters? Please write that number on your paper, label it "guess."

Question 2: Now use whatever tools you need, give me a better answer. I know some of you already know the formula for the area of a circle, but you may not use it unless you can tell me where it came from. (No one even tried.)

I can't tell you how happy I was to see all the different ways the the kids had tried to approximate area. Their perseverance humbled me. A few students drew triangles, knew to use "base times height divided by two," but erroneously used radius as height.

Last week I was reading Mimi's post about estimating area of circles, and Sue VanHattum reminded me of the rectangle model in her comment.

I had the kids fold their circles like this.

They cut out the pieces — turning every other piece 180 degrees — and glued them together. Cristian said, "First we had a circle, then triangles, then a rectangle. That's crazy!"

Never once did I answer any of the questions. I just asked them. I began with, "What is the area of this rectangle or parallelogram?" Step by step, as a class, the kids walked this equation all the way to Area = pi x radius^2.

This morning, two days after the activity, I did a My Favorite No to see if they remembered: 31 out of 33 students got the correct circumference formula; 24 of 33 got the correct area formula. Bonus if they showed me how the rectangle model helped explain the area of a circle.