My Other Math Sites
Lessons From the Classroom
A First Grade Lesson Using Vertical Whiteboards
As we wrapped up our book study of Peter Liljedahl’s Building Thinking Classrooms, and in my role as TOSA (teacher on special assignment) I do not have a classroom of my own, I asked one of our first-grade teachers if I may facilitate a thinking task using vertical whiteboards with her students.
To supplement the teachers’ classrooms with more whiteboard space, I reached out to Wipebook for durable dry-erase chart paper surfaces (with grids on one side!). The reusable Flipcharts they sent were the perfect tool to use over her glass doors and windows! They can easily be removed and reused as many times as you want — an eco-friendly solution to reduce paper waste. Our district has also purchased whiteboard easels with adjustable heights.
The teacher already had her groups paired up with their “thinking partners.” Each whiteboard space was labeled such as “Blue 1 and 2” so the students knew where they would be working.
We had the students sitting on the carpet area for me to launch the task. I taped this flower image on the board and asked how many circles they saw. They said seven.
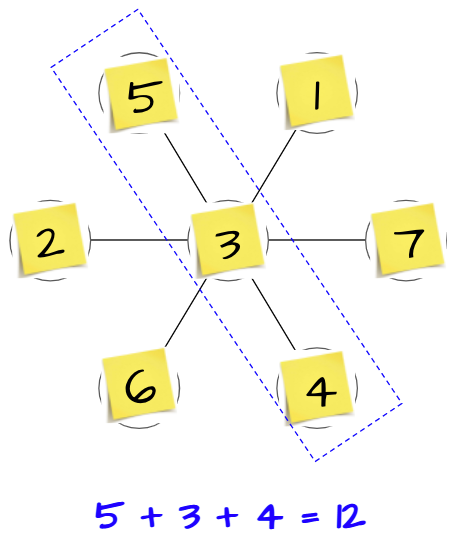
Then, I counted out along with them seven sticky notes, marked from 1 to 7. This great Flower Petal Puzzles thinking task is from Dan Finkel, founder of Math for Love. The intent of the task was for students to write the numbers inside the circles/petals and to keep track of numbers they have used by crossing them off at the bottom of the page. Dan also suggested using counters. However, I wanted the students to do these vertically, so counters would not work, and writing numbers in and erasing them is challenging and cumbersome, especially for young students. The use of sticky notes was really key in helping students stay focused on the task because it was easy to swap out numbers and keep their brains moving.
I called up one student at a time to place a numbered sticky note into an empty petal without further instruction or stating what the goal was. When there was only one space left, I said it was my turn and placed the number in. I wish I had taken a picture of the board at this point. But since I didn’t, below is a picture of the flower with randomly placed numbers. I then told them these three petals are connected and asked them for the sum and wrote the equation.
We found the sum of the other two groups.
It was not until then that I told them the challenge of the task was to have all three sums be the same. And that the sum is 10. So off they went with their thinking partners to their whiteboards!
Sure enough one pair got the solution within 5 minutes, and we asked them to keep their equations (not erase them), and to do the next challenge which was to get a sum of 12 for all three equations. Some time later, all of the groups except for two got the 10 puzzle. We came over and applauded them for their perseverance and asked if they would like a clue. They nodded and we let them know to place the number 1 at the center of the flower. When this group got the solution with the hint, the girl excitedly said to me, “This is a lot of fun!”
I tweeted this out later as truly it was the best hour with first graders for me. So much perseverance, so much thinking, so much fun. The groups were in different stages (finding sums of 10, 12, and 14), and two groups ended the hour working on the “ultimate challenge” of creating their own puzzle using a 9-petaled flower.
Updated 04/11/2022
I just thought of a way to take this 1st grade task to next level. https://t.co/7vQzKk4kuP
— Fawn Nguyen (@fawnpnguyen) April 1, 2022
7 circles: possible sums r 10, 12, 14 (what #s go in middle circle of these sums, respectively?)
What r possible sums for n (odd) circles? How many equations [of equal sum] for each n?
Joint Investment
Would you have known immediately that the number 1729 is the sum of two cubes?
You think you're so smart, but you're no Ramanujan. He told Hardy that 1729 is the sum of two cubes in two ways: 1³+12³ and 9³+10³.
I read The Man Who Knew Infinity a few years ago. I might check out the movie when it hits a theatre near me. Like Netflix.
Also, I subjected my students to this 14-minute podcast about Hardy and Ramanujan.
One more "fair share" task for my 8th graders from Peter Liljedahl's site because I like it and don't want to work in the textbook as our students are taking the Smarter Balanced Tests this week.
Joint Investment
Six years ago you made an investment with a friend – you bought a house together. It wasn’t only an investment, it was also a favor. Your friend didn’t have a place to live and didn’t have enough money to buy a house. So, you pooled your money and bought a $300,000 house for your friend to live in. You provided $50,000 for the down payment and your friend provided $25,000. Because of the size of the down payment it meant that the mortgage was relatively low – only $1000 a month – which your friend paid. During the six years all property tax payment were split evenly between you as were all major renovations and upgrades. Well, it is now six years later and your friend is getting transferred to Ontario. So, you have sold the house for $500,000 (the market has been good to you). There is still $200,000 outstanding on the mortgage. How will you split the $300,000 equity between you? Justify your decision.
Two groups of students have submitted their solutions thus far.
…
…
…
…
…
…
…
…
…
…
The Shoe Sale
This problem is from Peter Liljedahl's site.
The Shoe Sale
You decide to take advantage of a buy 2 pair get 1 pair of equal or lesser value for free sale at the local shoe store. The problem is that you only want to get two pairs of shoes. So, you bring your best friend with you to the store. After much deliberation you settle on two pairs of shoes – a sporty red pair for $20 and a dressy black pair for $55. You friend finds a practical cross trainer for $35. When you proceed to the check out desk the cashier tells you that your bill is $90 plus tax (the $20 pair are for free). How much should each of you pay? Justify your decision.
Peter lists this problem under "Senior High School (10-12)." I give it to both my 6th and 8th graders. I like this problem because I like hearing how kids think about "fair sharing." A few 6th graders think each person should pay $45. I don't think these kids have too many friends. (That was mean.)
One of my 6th graders says one person should pay 2/3 of the $90 and the friend pays 1/3. But her answers are $59.40 and $29.60, respectively. My math says 2/3 of 90 is 60, so I call her up to explain. She has her calculator in hand, and I see her punch in .66 while mouthing "two thirds."
It was an opportunity for me to yell and scream at the children for turning a perfectly good number of 2/3 into mush.