Students Embroiled in Conway's Rational Tangles

Me: We're going to do an activity today.

Jonathan: Let me guess. It's your favorite activity.

M: How did you know that?

J: You say that about everything we do.

M: You have a problem with that?

J: No. But I've lost track of how many favorites you have.

M: And I've lost track of how many times you've talked without permission.

M: But I still love you though.

J: I love you too, Mrs. Win.

Yeah, so that was the exchange we had at the start of Algebra. Kids are used to me telling them how much I love them because I really do. It's the Monday-through-Friday kind of love that lasts from 8:25 am to 2:50 pm — at which time I say to them, "Get out of my room." They laugh at this; they must think I'm kidding.

Tom Davis did John Conway's Rational Tangles with us at last summer's Math Teachers' Circle (MTC). Reading this post (with lots of solid links) over the weekend reminded me of this fun lesson.

I haven't come across a written lesson of this done with school children though. So, here I am. It's my new favorite lesson.

Day 1

I use the two ropes given to us from the MTC training.

I ask for 4 student volunteers to come up front. The rest of the class needs to pay careful attention and record in their math journals what I record.

Important

1. The two ropes can be of the same color. Two colors are used in practice so it's easier for students to follow along.

2. The 4 people holding the robes can be identical quadruplets — who they are doesn't matter, but I'm referring to them as colored dots if and only if they move or change position.

3. The letters A, B, C, and D refer to the 4 positions, like goalposts, therefore they do not change.

The starting position is when the two ropes are horizontally parallel, and this state has a value of 0.

There are only TWO "moves" in this game of rational tangling and untangling.

A TWIST is when people in positions A and C switch places. Green moves underneath red's rope. 

This move equals plus 1, meaning our ropes went from a value of 0 to a value of 1.

The only other move is called ROTATE. A rotate is when the 4 people move in a clockwise direction, each person taking the space previously occupied by the person to his/her left.

Now, the money question to the kids: What does the operation "rotate" do to the value of the ropes? 

We know a twist = +1, but a rotate = ??

So, in the diagram just above, our ropes went from a value of 0 to what value?

The kids want to do a twist after this rotate, but they realize that the ropes are now funny — or vertically parallel — that you can't do a twist since there's no rope for yellow to go under, and green and yellow switching places does not change how the ropes look.

Because a twist can't be done, our only other choice is to rotate — which leads us to this configuration.

AHA! This brings us back to 0 because the ropes are horizontally parallel again.

Symbolically, we can record what happens with 2 rotates (R), done back to back, when we start with 0: 0 (R) -> ? (R) -> 0.

We need to test this again, so let's start at 0. Do 1 twist (T), then 2 rotates. This means the value goes from 0 to +1, then to some unknown value after the first rotate, but after the second rotate, the ropes have a value of +1 again.

0 (T) -> 1 (R) -> ? (R) -> 1

Someone suggests that the rotate operation means multiply by -1. This is a lovely suggestion as it seems to work: a twist of 0 makes it +1, then if a rotate means multiply by -1, then we have -1, then another rotate makes it +1 again.

Then we try to do 2 twists from 0, giving us +2. So if we do a rotate now, our value should be -2. This means we can then do 2 twists to add 2, bringing us back to 0. But it did not work!

We do a few more test moves. Someone suggests a rotate subtracts 2. Others suggest that it throws a negative sign on the value somehow, somewhere. The kids are struggling. One claims a massive headache from all this, to which I say, "Enjoy that, honey."

We have a few conjectures though:

1. Starting from 0 and doing 1 rotate puts our ropes in a weird (undefined?) state where we can't do a twist after that.

2. Two rotates, back to back, bring the ropes back to the same state prior to the first rotate. Therefore, we should not do 2 consecutive rotates unless we just want to waste time.

3. A rotate must involve a negative — how else can we get back to 0 by twisting alone?

I instruct the 4 volunteers through this entire sequence of moves below. The class and I record each step. As soon as we put in a rotate, however, and not know exactly what this move does to the value, we just have to put question marks in all these spaces.

I can't take pictures of the kids doing this while also teaching them, so I've re-created this at home with a shoe box and 4 well-behaved binder clips that act has the rope holders.

Step 1: Start at 0.

Step 2: Do 1 twist. Value now is 1.

Step 3: Another twist. Value = 2.

Step 4: Another twist. Value = 3.

Step 5: Rotate. Value = ??

Step 6: Twist. Value = ??

Step 7: Rotate. Value = ??

Steps 8 & 9: Twist and twist again. Value = ??

Step 10: Rotate. Value = ??

Step 11: Twist. Value = ??

Step 12: Twist. Value = 0

Kids realize that if the last two twists got the ropes back to 0, then Step 10 must have a value of -2, and Step 11 is -1.

After about 20 minutes of doing the dance with the ropes and agonizing, a couple of geometry kids figured out what a rotate does to the value of the ropes. Algebra kids did not have enough time, so they'll continue their investigation on Day 2. Because I only see my 6th graders one period a day (instead of 2 periods/day for algebra and geometry) and they being younger, I told them what "rotate" does mathematically. There will still be plenty of math for them to do on Day 2.

Day 2

It's more fun when the kids can participate instead of just watching the 4 kids in front of the room. I go to Lowe's to pick up the ropes for about $12.

Kids will be in groups of five, four are holding the ropes and doing the moves, one person records and oversees that everyone in group is moving appropriately. Each group gets 2 ropes, one of each color, and each rope is about 7 feet long.

All the groups will first follow the same set of crazy tangle moves that I give them. Something like, "Start at 0, twist 4 times, rotate, twist 2 times, rotate, twist 3 times, rotate, twist 5 times. Okay, now figure out how to get back to 0."

(Algebra kids still need to figure out what a rotate does to the value of the ropes before they break out into groups.)

When they figure out my tangle, I'll have each team create their own tangle and undo the mess. Of course they'll have to record all the moves from 0 and back to 0 symbolically.

How will your kids figure out what the "rotate" operation does?

Update 03/13/12

As planned, I had the kids in groups of 5 and made this tangle: Start at 0, twist 3 times, rotate, twist twice, rotate, twist 5 times. Then they had to untangle this and get back to 0.

How the kids persevered through this entire problem was really amazing. 

They hugged each other and jumped for joy when they untangled the ropes. Especially touching was the group that was the last to solve the problem — wish I'd captured it on camera/video.

This group was first to find the solution. (They had to create one of their own after this.)

If above video is not enough to convince you to do this with your kids, here's a tweet from Sam this morning. Imagine that — this activity blew young Sam's brilliant mind.

Math Teachers' Circle

Last Monday morning I was on a SuperShuttle from SFO to the Creekside Inn. But I was running a little late, so the driver took me straight to the American Institute of Mathematics (AIM). I was expecting a tall building with lots of glass windows — instead the driver stopped here.

I was at AIM to attend a one-week workshop on How to Run a Math Teachers’ Circle. I stumbled upon this opportunity simply by doing a search for a Circle that I could be a participant in. But there wasn't one in my area that I knew of, hence my always-ambitious-but-never-know-what-the-hell-I'm-getting-myself-into evil twin said, Why don't you start one?

I asked Erin, my next-door math colleague, to join me. She and I fulfilled the requirement for "two middle school math teachers" — we just needed to find "two mathematicians and one administrator or organizer" to make a team of five.

I contacted Brianna Donaldson, AIM's Director of Special Projects, to help us round out the team. Within the week, she hooked us up with Nate and Hala, both are math professors at Cal Lutheran. We still needed a fifth member, so I asked Melissa, another math colleague (there are only 3 of us at our junior high) — she graciously got on board.

Needless to say I was thrilled to hear that our team was chosen among many (like hundreds of thousands of teams) to receive full funding to participate in this workshop.Below is a summary of my week in beautiful Palo Alto, California. (For some reason it's easier for me to write in present tense when I recount a story — maybe it's an ELD or ESL thing.)

Monday

I meet the other two members of our team for the first time, Nate and Hala. I've seen their pictures online, and they look the same — thank God!There are six teams: 2 from Texas, 1 Kansas, 1 New York (Rochester), and 2 California.

Josh Zucker appears first on the agenda, "Introduction to Problem Solving." I've always wanted to meet Josh — his name appears on most of the cool math problems that I first encountered at the Julia Robinson Mathematics Festival in Los Angeles. Turns out he's the director.

He facilitates this classic problem:

The numbers 1 through 100 are written on the board. You choose any two numbers x and yand erase them, writing xy + x + y in their place. You continue to do this until one number remains. What are the possible values for that remaining number?

I just want to post the problems here without further discussions on them so that you — the thinker, the mathematician, the teacher, the student, the problem solver — get to struggle with the problem and construct meaning for yourself.

There are three things that happen consistently each day, so I'll just mention them once here:

1. We get two hours (TWO HOURS!!) for lunch as the nearby restaurants are about a 15-minute walk away.

2. After lunch, we work within our group on logistics and fundraising to run our own math circle.

3. "Happy Hour" greets us at the end of each session, if we wish to stay.

Tuesday

Tatiana Shubin is a math professor at San Jose State University; she presents Grid Power. I've always required my students to use quadrille-ruled composition notebooks to take notes and such, but after hearing Tatiana speak, I want my kiddos to do ALL their math work on grid paper!

Tatiana gives us this delightful problem:

How many squares are there in a 7 x 7 square?

Paul Zeitz introduces us to "Mathematical Games." He's a math professor at the University of San Francisco and is taking a sabbatical this year. I've ALWAYS wanted to buy the book The Art and Craft of Problem Solving — lo and behold, Paul is the author! But Paul doesn't once mention his book. (I made the connection after the workshop was over.) Instead he recommends James Tanton's Solve This. I need these books.

Two of the games we work on:

Takeaway. A set of 16 pennies is placed on the table. Two players take turns removing pennies. At each turn, a player must remove between 1 and 4 pennies (inclusive). The winner is the last player to make a legal move.

Puppies and Kittens, aka Wythoff's Nim. We start with a pile of kittens and puppies. Two players take turns; a legal move is removing any number of puppies or any number of kittens or an equal number of both puppies and kittens. The winner is the last player to make a legal move.

I really like how Paul keeps track of winning/losing moves as oasis/desert points on a horizontal line. So with the two variables like in Puppies and Kittens, we use a coordinate plane instead to record.

Wednesday

Diana White is a math professor at the University of Colorado Denver. Diana facilitates us through the Exploding Dots problem. (James Tanton owns the dots.)

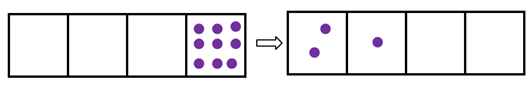
Say you have a machine that holds ping pong balls. If you put three balls in the far right slot, they'll explode and two balls will move one space left into the next slot. Like this:

This happens with any set of three balls in one slot, therefore the explosion continues until there are fewer than three balls per slot. Thus, starting with 9 ping pong balls, the result looks like this:

Tom Davis is a retired math professor; he walks us through Conway's Rational Tangles. I don't even know how to begin to explain and illustrate this problem. It requires four students and two long ropes — each student holding one end of a rope. The task is to do two (and only two) commands of "twist" and "rotate" to tangle up the ropes — the challenge then is to untangle the ropes in a systematic way that involves arithmetic with positive and negative fractions. Okay, my explanation is as clear as mud.

The point is it is a wonderful activity that kids and teachers will absolutely love to get their hands on, literally. The other point is I'm better than you because I have the two ropes. See?

Thursday

Paul Zeitz is back this morning with "How to Gamble If You Must." We play a few dice games, then we work on Two Lottery Tickets:

It costs a consumer $1 to buy a Klopstockia lottery ticket. The buyer then scratches the ticket to see the prize. Compute, to the nearest penny, the expected profit that the state of Klopstockia makes per ticket sold, given the following scenarios for prizes awarded. (The state will make a profit if the expected value to the lottery ticket is less than $1.)

Friday

There is no math activity on our last day. Each team has 10 minutes to present the how/what/where/when of their own future Math Teachers' Circle. We each get a T-shirt and our team pictures taken. We bid farewell at noon.

I'm grateful to everyone who made this workshop possible. And I love my team!! So I lied about us being chosen among hundreds of thousands. I had never drank so much wine in one week. I took two wine corks home to practice a trick Josh had taught us. Please consider joining a Math Teachers' Circle in your area. Our team leads the Thousand Oaks MTC and would love to have you. (I'm working on our website.) We owe it to our students to make math engaging and accessible.